Skip to main content
   
Logo image

Physics Bootcamp

Samuel J. Ling

Section 33.7 Dielectrics Bootcamp

Exercises Exercises

Electric Dipoles

1. Polarization of Carbon Atom in External Electric Field.
Follow the link: Exercise 33.1.4.1.
3. Polarization of Water Molecule and Charges on Each Atom.
Follow the link: Exercise 33.1.4.3.

Force and Torque on Electric Dipoles

4. (Calculus) Force and Torque on a Dipole in a Non-uniform Field.
Follow the link: Example 33.7.
5. (Calculus) Computing Force on a Dipole by a Point Charge.
Follow the link: Example 33.8.
6. Torque on Electric Dipole of Water by External Electric Field.
Follow the link: Exercise 33.2.3.1.

Potential Energy of Electric Dipoles in an External Field

7. Energy for Flipping a Dipole Upside Down.
Follow the link: Example 33.10.
8. Electrostatic Energy of a Dipole in the Presence of a Point Charge.
Follow the link: Exercise 33.3.2.1.

Electric Potential of a Dipole

9. Force Between an Electric Dipole and a Point Charge.
Follow the link: Example 33.14.
10. Force on a Point Charge from an Electric Dipole.
Follow the link: Example 33.15.
11. Torque on a Dipole from Field of another Electric Dipole.
Follow the link: Example 33.16.

Linear Dielectrics

12. Electric Field in Paper Between Two Charged Paper Plates.
Follow the link: Exercise 33.5.6.1.
13. Polarization of Plastic Wrapped Around a Charge Steel Sphere.
Follow the link: Exercise 33.5.6.2.
14. Electric Field and Polarization of Two Different Layers of Dielectrics Filling Space Between Parallel Plates.
Follow the link: Exercise 33.5.6.3.

Miscellaneous

15. Force on a Dielectric Slab Between Two Charged Plates.
Follow the link: Example 33.22.
16. Torque on Ammonia Molecule and Energy for Flipping the Orientation of Dipole Moment.
An ammonia molecule has an electric dipole moment of \(5.0 \times 10^{-30}\) C.m. It is placed in an external electric field of \(1,000\) V/m. (a) What is the torque on it at the time the dipole is pointed at a \(60^{\circ}\) angle with respect to the electric field? (b) Once aligned, how much energy will it take to flip the orientation of the dipole by \(180^{\circ}\text{?}\) Write your answer in the electron volt (eV) unit. The unit \(1\) eV = \(1.6\times 10^{-19}\) J.
Hint.
Use definitions
Answer.
(a) \(4.3\times 10^{-27}\) N.m, (b) \(6.3\times 10^{-8}\) eV.
Solution 1. a
The torque on a dipole is equal to the vector product of the dipole moment and the electric field. Therefore, the magnitude of the torque will be
\begin{equation*} \tau = p\:E\:\sin\theta = 5.0\times 10^{-30}\:\textrm{C.m}\times 1000\:\dfrac{\textrm{V}}{\textrm{m}}\:\sin 60^{\circ} = 4.33\times 10^{-27}\:\textrm{N.m}. \end{equation*}
The direction of the torque vector can be obtained by using the right-hand rule. therefore, if you place the vector \(\vec p\) towards the positive \(x\)-axis and vector \(\vec E\) in the \(xy\)-plane towards a direction in the space \(y>0\text{,}\) then the torque will be pointed towards the positive \(z\)-axis.
Solution 2. b
Flipping of a dipole’s direction requires energy equal to \(2pE\text{.}\)
\begin{equation*} U = 2pE = 2\times 5.0\times 10^{-30}\:\textrm{C.m}\times 1000\:\dfrac{\textrm{V}}{\textrm{m}} = 1.0\times 10^{-26}\:\textrm{J}. \end{equation*}
17. Torque and Energy of a Water Molecule in External Electric Field.
A water molecule is \(500\, \text{nm}\) from a point charge A (\(2\, \text{fC}\)). (a) Find the electric force on the water molecule when the dipole moment of the water molecule is pointed towards the charge. (b) How much energy will it take to rotate the water molecule so that dipole moment faces away?
Solution 1. a
Note that the electric field of the point charge is not uniform. Therefore, there will be a force on the dipole given by the formula for the force on a dipole in an inhomogenous field. The magnitude of the force will be given by
\begin{align*} F \amp = \dfrac{1}{4\pi\epsilon_0}\:\dfrac{2pq}{r^3}\\ \amp = 9\times 10^9\:\dfrac{2\times 6.1\times 10^{-30}\times 2\times 10^{-15}}{\left(500\times 10^{-9}\right)^3} = 1.8\times 10^{-15}. \end{align*}
Solution 2. b
The energy will be given by \(2 p E\text{.}\)
\begin{align*} U \amp = 2 p E = 2\times 6.1\times 10^{-30}\:\textrm{C.m}\times \left[ 9\times 10^9\:\dfrac{2\times 10^{-15}}{\left(500\times 10^{-9}\right)^2} \right]\: \textrm{N/C}\\ \amp = 8.8\times 10^{-22}\:\textrm{J}. \end{align*}
18. Analyzing a Capacitor with Two Dielectrics Filling the Space Between Plates.
A parallel plate capacitor is charged so that positive plate has a charge density \(+\sigma\) on the side facing the negative plate which has charge density \(-\sigma\) on the side facing the positive plate. The plates are separated by a distance \(d\) and area of one side of the each plate is \(A\text{.}\)
The space between the plates is filled with two slabs made of dielectric materials with dielectric constants \(\epsilon_{r1}\) and \(\epsilon_{r2}\) respectively of thickness \(d/2\) each (Figure 33.25). (a) Find the electric field in each slab. (b) Find the potential difference between plates. (c) Find the capacitance of the capacitor. (d) Find the polarization P of each dielectric slab.
Figure 33.25.
Answer.
(c) \(\frac{1}{C} = \left( \frac{1}{\epsilon_{r1}} + \frac{1}{\epsilon_{r1}} \right)\frac{d}{2A\epsilon_0}\text{.}\)
Solution 1. a
The magnitude of the electric field of the charges when there is no dielectric between the plates is
\begin{equation*} E_0 = \dfrac{\sigma}{\epsilon_0}. \end{equation*}
Therefore, the magnitudes of the electric field at the space points in the two slabs are
\begin{equation*} E_1 = \dfrac{E_0}{\epsilon_{r1}},\ \ \ E_2 = \dfrac{E_0}{\epsilon_{r2}}. \end{equation*}
Solution 2. b
The potential difference between the plates is obtained by integrating the electric field as usual,
\begin{equation*} \Delta V = -\int _{(-)}^{(+)}\: \vec E\cdot d\vec r = E_1\:\dfrac{d}{2} + E_2\:\dfrac{d}{2} = \left( \dfrac{1}{\epsilon_{r1}} + \dfrac{1}{\epsilon_{r2}} \right)\: E_0\:\dfrac{d}{2}. \end{equation*}
Solution 3. c
Writing \(E_0\) in terms of \(Q\) on the plate we obtain the following for \(\Delta V\text{.}\)
\begin{equation*} \Delta V = \left( \dfrac{1}{\epsilon_{r1}} + \dfrac{1}{\epsilon_{r2}} \right)\: \dfrac{Q}{A\epsilon_0}\:\dfrac{d}{2}. \end{equation*}
Now, we can deduce the capacitance \(C\) of the structure from the ratio \(Q/\Delta V\text{.}\)
\begin{equation*} C = \dfrac{2\epsilon_0\:A}{\left( \frac{1}{\epsilon_{r1}} + \frac{1}{\epsilon_{r2}} \right)\: d}. \end{equation*}
Solution 4. d
The magnitudes of the polarization of the two slabs are obtained by the electric field in the two slabs.
\begin{align*} \amp P_1 = \epsilon_0\:(\epsilon_{r1}-1)\:E_1 = (\epsilon_{r1}-1)\:\dfrac{\sigma}{\epsilon_0 \epsilon_{r1}} = \left(1-\dfrac{1}{\epsilon_{r1}} \right) \: \sigma \\ \amp P_2 = \left(1-\dfrac{1}{\epsilon_{r2}} \right) \: \sigma \end{align*}
19. Capacitor Filled With Two Dielectrics Each Spanning the Space and Occupying Half of Space.
The plates of a parallel plate capacitor are separated by a distance d and area of one side of the each plate is A. The space between the plates is filled with two slabs made of dielectric materials with dielectric constants \(\epsilon_{r1}\) and \(\epsilon_{r2}\) respectively each of thickness d and occupying half of space (Figure 33.26). Find the capacitance.
Figure 33.26.
Answer.
\(C = \frac{A\epsilon_0}{d}\left( \frac{\epsilon_{r1} + \epsilon_{r2}}{2} \right)\text{.}\)
Solution.
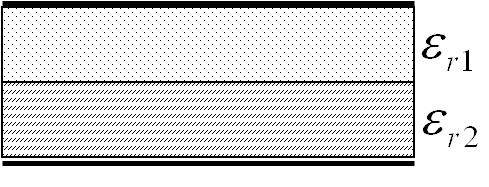
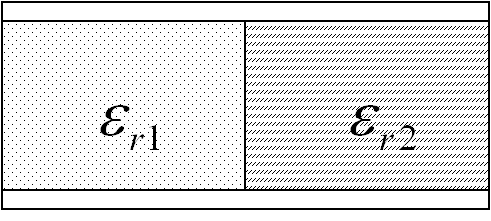
Note that although the potential difference between the plates is same between any two points of the two plates, the charges on the plates are not uniformly distributed - there will be a different charge density on the plates in the area of \(\epsilon_{r1}\) than in the area of \(\epsilon_{r2}\text{.}\) Let \(\sigma_1\) and \(\sigma_2\) be the corresponding surface charge densities in the two regions of the plates. The charge conservation tells us that
\begin{equation*} \sigma_1 \:\dfrac{A}{2} + \sigma_2\: \dfrac{A}{2} = \sigma\:A,\ \ \Longrightarrow\ \ \sigma_1 + \sigma_2 = 2\sigma.\ \ \ \ (1) \end{equation*}
The magnitudes of the electric field between the plates in the two regions occupied by the two different dielectrics will be
\begin{equation*} E_1 = \dfrac{\sigma_1}{\epsilon_{r1}\:\epsilon_0},\ \ E_2 = \dfrac{\sigma_2}{\epsilon_{r2}\:\epsilon_0}. \end{equation*}
Now, when we calculate the potential difference between the plates using these two different expressions for the electric field we should get the same value. \begin{align*} \Delta V = \dfrac{\sigma_1\: d}{\epsilon_{r1}\:\epsilon_0}\ \ (\textrm{using } E_1),\\ \Delta V = \dfrac{\sigma_2\: d}{\epsilon_{r2}\:\epsilon_0}\ \ (\textrm{using } E_2) \end{align*} Equating the two we get
\begin{equation*} \dfrac{\sigma_1\: d}{\epsilon_{r1}\:\epsilon_0} = \dfrac{\sigma_2\: d}{\epsilon_{r2}\:\epsilon_0},\ \ \Longrightarrow\ \ \sigma_2 = \dfrac{\epsilon_{r2}}{\epsilon_{r1}}\: \sigma_1.\ \ \ (2) \end{equation*}
Putting (2) into (1) helps us solve for \(\sigma_1\) with the result
\begin{equation*} \sigma_1 = \dfrac{2\epsilon_{r1}}{\epsilon_{r1} + \epsilon_{r2}}\: \sigma.\ \ \ \ (3) \end{equation*}
Put (3) into either of the two expressions for \(\Delta V\) to get
\begin{equation*} \Delta V = \dfrac{2\sigma d}{\epsilon_0\: (\epsilon_{r1} + \epsilon_{r2}) } = \dfrac{2Q d}{A\epsilon_0\: (\epsilon_{r1} + \epsilon_{r2}) }. \end{equation*}
The ratio \(Q/\Delta V\) gives the capacitance \(C\) to be
\begin{equation*} C = \dfrac{\epsilon_0\: A}{d} \left( \dfrac{\epsilon_{r1} + \epsilon_{r2}}{2} \right). \end{equation*}
20. Space Between Plates of a Capacitor Filled with Three Dielectrics.
A parallel plate capacitor has plates of area 100 \(cm^2\) and separation of plates 3 mm. The gap between the plates is filled with equal thickness (1 mm each) of layers of mica, paper and a material of unknown dielectric constant. The capacitance is then measured with a capacitance meter and found to be 200 pF. What is the dielectric constant of the unknown material?
Answer.
\(19\text{.}\)
21. Force on Dielectric Oil Pulling Oil Between Plates of a Cylindrical Capacitor.
Consider a cylindrical capacitor that has a metal cylinder of radius \(R_1\) in the middle surrounded by a metal cylindrical shell of inner radius \(R_2\text{.}\) Assume the capacitor to be very long.
It is dipped in a large tank of motor oil of dielectric constant \(\epsilon_r\) so that a height \(D\) of the capacitor is under oil. When the inside and outside of the capacitor are connected to constant voltage source of \(V\) volts it is found that gasoline rises to a height \(h\text{.}\) Find the value of \(h\) in terms of other quantities. Assume the density of motor oil to be \(\rho\text{.}\) Note that a voltage source maintains the voltage difference between its terminals.
Figure 33.27.
Solution.
For reference of directions, let \(y\)-axis be pointed up with origin at the bottom of the cylindrical capacitor. If the space between the capacitor plates is filled to a height \(y\text{,}\) then the capacitance will be
\begin{equation*} C = \left[ \dfrac{2\pi\epsilon_0\epsilon_r}{\ln(R_2/R_1)} \right] y + \left[ \dfrac{2\pi\epsilon_0}{\ln(R_2/R_1)} \right](L-y), \end{equation*}
where \(L\) is the total length of the capacitor. As the oil climbs the potential between the plates will change for the same \(Q\) on the plates. Therefore, let us write the energy in terms of \(Q\) first which we can change to \(CV\) at the end.
\begin{equation*} U(y) = \dfrac{1}{2}\: \dfrac{Q^2}{C(y)}. \end{equation*}
Therefore, the \(y\)-component of the electrostatic force on the oil will be
\begin{equation*} F_y = - \dfrac{\partial U}{\partial y} = \dfrac{1}{2} V^2 \left[ \dfrac{2\pi\epsilon_0\epsilon_r}{\ln(R_2/R_1)} - \dfrac{2\pi\epsilon_0}{\ln(R_2/R_1)} \right]. \end{equation*}
This force will be balanced by the weight of the oil when the height is \(y=h\text{.}\)
\begin{equation*} \dfrac{1}{2} V^2 (\epsilon_r-1)\dfrac{2\pi\epsilon_0}{\ln(R_2/R_1)} = m\: g = \pi (R_2^2 - R_1^2)\: h\: \rho\: g. \end{equation*}
This gives the following for \(h\text{.}\)
\begin{equation*} h = \dfrac{ \epsilon_0 (\epsilon_r-1)\: V^2}{\pi (R_2^2 - R_1^2)\: \rho\: g\ln(R_2/R_1)}. \end{equation*}
PrevTopNext
Found typo?