Just as any mechanical wave, sound wave transports energy and momentum from the source to the detector, not by any transport of matrial, but by coupling of motion that causes the wave to travel.
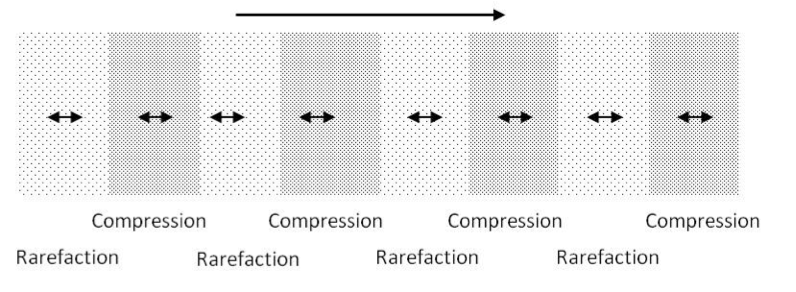
We saw in the last chapter that mechanical waves on a string had vibrations perpendicular to the string along which the wave travelled. But, in the case of sound in air, the particles of air vibrate along the same line as the travel of the sound wave. That is, sound in air is longitudinally polarized (see Figure 15.1).
Figure15.1.Propagation of sound in air illustrated by having regions of compression and rarefaction of air particles. The compressed regions correspond to higher pressure than the ambient pressure and the rarified regions have lower pressure.
When sound travels through air, particles of air vibrating in the forward direction press against other particles of air creating a higher pressure than when the wave was not there. This gives rise to regions of compression and rarefaction of air. That means, we can alternately speak of sound as pressure differential wave rather than particle vibration wave.
As a matter of fact sound wave in all fluids are longitudinal since fluids cannot provide restoring force to a shear stress generated when a sound wave traveling in the medium. Sound waves in solids can be both longitudinal and transverse. You will study various types of stress in another chapter.
Both light and sound have (1) physically measurable characteristics and (2) human-perceived characteristics. For instance, frequency and intensity of sound can be measured by instruments which provide objective measures of the wave. However, we also have measures based on uman perception - perception of frequency is called pitch and perception of intensity is called loudness which can be subjective and perception may vary from one person to another.
Generally, higher frequency sound is perceived to be at a higher pitch than the sound at lower frequency. But, our brain can sometimes interpret louder sound to be at a higher pitch even though it may have the same frequency. Sound of higher intensity is generally perceived as louder. But that also depends on the frequency. We Will discuss the human response in another place.
If we have a sinusoidal sound wave, it will have a single frequency \(f\) and a single wavelength \(\lambda\text{.}\) They will be related to speed \(v\) by the fundamental formula of wave motion by
\begin{equation}
v = f \lambda.\tag{15.1}
\end{equation}
This simply says that wave travels a distance equal to the wavelength in time \(1/f\text{.}\)
By analyzing the vibration of the particles of the medium, we can show that the speed of mechanical waves, including sound wave, through a medium comes from a competition between two opposite tendencies - a restoring force whose tendency is to bring the particle to equilibrium and an inertia whose tendency is to maintain the motion. In a one-dimensional system such as a string, the restoring force is provided by the tension in the string \((F_T)\) and the inertia is provided by mass per unit length of the string \((\mu )\text{.}\) The speed of mechanical wave in a string was stated in the last chapter to be
\begin{equation*}
v =\sqrt{\frac{F_T}{\mu}}.
\end{equation*}
The speed of sound in air is similarly related to the properties of air. The restoring force is provided by the bulk modulus \(B\) and the inertia is provided by mass per unit volume \(\rho\text{.}\) The speed of sound in air is therefore given in terms of properties of air by the following.
\begin{equation*}
v = \sqrt{\frac{B}{\rho}}.
\end{equation*}
The density of air is not constant. It depends on the temperature and pressure. We quote here experimental relation of dependence of speed on temperature. At 1 atm and \(0^{\circ}\text{C}\text{,}\) the speed of sound in air is found to be 331 m/s and at another temperature \(t^{\circ}\text{C}\text{,}\) the speed of sound in air at 1 atm is given by the following approximate formula.
Thus at room temperature of \(20^{\circ}\text{C}\text{,}\) the speed of sound in air is approximately \(343\text{ m/s}\text{.}\)
Speed of sound is different in different materials depending upon their bulk moduli and densities and the polarization of the wave. While we have only longitudinally polarized waves in liquids and gases, sound waves in solids can be also transverse. Table 15.2 gives the speed of sound in some common materials of interest.
Table15.2.Speed of sound in various material 1
at \(25^{\circ}\text{C}\) and \(1\text{ atm}\)
2
Source: Kaye and Laby, Table of physical and chemical constants 16th edition (published 1995)
Speed of
Speed of
Medium
longitudinal wave
transverse wave
(m/s)
(m/s)
Isotropic Solids
Aluminum, rolled
6374
3111
Brass
4372
2100
Polycarbonate
2220
910
Pyrex Glass
5640
3280
Steel, Stainless
5980
3297
Liquids
Blood
1584
Glycerin (Glycol)
1920
Mercury
1449
Water, Distilled
1496
Water, Sea (3.5% salinity)
1534
Gases
Air
343
Helium (\(0^{\circ}\text{C})\)
972
Hydrogen (\(0^{\circ}\text{C})\)
1286
Subsection15.1.1Sound through Solid Media
Since sound is a mechanical vibration, it can travel through any material medium. In liquid and gas, only longitudinal sound is possible because fluids do not have a restoring force in tangential direction, they have restoring force only against compression. Solids have restoring force for compression as well as shear forces. Therefore you will find three polarizations of sound waves in solid: one longitudinal, and two transverse modes, one for each perpendicular direction.
The speeds of longitudinal and transverse waves are different since the restoring forces are different for them.
\begin{equation*}
v_{\text{sound}} = \sqrt{\frac{\text{Restoring force per unit area}}{\text{Inertia as given by density}}}
\end{equation*}
For a uniform isotropic material the two transverse waves have the same speed. Let \(Y\) be the Young’s modulus, \(G\) the shear modulus, and \(\rho\) the density of the solid. The speed of longitudinal sound \(v_L\) is related to the Elastic modulus \(E\text{.}\)
where \(E = Y+\frac{3}{4} B\text{.}\) Here \(Y\) is the Young’s modulus and \(B\) the bulk modulus. On the other hand, the speed of transverse waves \(v_T\) is related to the shear modulus.
For instance, steel has \(Y\approx 215\text{ GPa}\text{,}\)\(B = 166\text{ MPa}\text{,}\)\(G\approx 84\text{ GPa}\text{,}\) and density \(7,800\text{ kg/m}^3\text{,}\) therefore the longitudinal and transverse waves travel at different speeds in steel.
The numbers here are a little different than those listed in the table because of the temperature dependence of sound. In general, shear stress \(G\) is less than the Young’s modulus \(Y\text{.}\) Hence speed of transverse waves will be less than that of longitudinal wave. Sound waves in solids are used to find defects inside solid materials by non-destructive means. The non-destructive techniques based on propagation of waves in material media have important applications in medical physics and other engineering fields. For instance, in aeronautics, invisible cracks in the wings of air planes can be detected even before they become large enough to cause an accident.
Exercises15.1.2Exercises
1.Speed of Mechanical Wave in Air.
Find the speed of a mechanical wave in air of density \(1.2\, \text{kg/m}^3\) and bulk modulus \(10^5\,\text{N/m}^2\text{.}\)
A sonar is an ultrasound device that is used to map the surface of the ocean. At a particular place the echo is heard 2 seconds after the sonar sends an ultrasound. How deep is the ocean there? Use 1500 m/s for the speed of sound in salt water.
Answer.
\(1500\ \text{m}\text{.}\)
Solution.
Let \(D\) be the depth and return time be \(T\text{.}\) The sound must travel \(2D\) to return in time \(T\text{.}\)
4.Speed and Wavelength of Ultrasound Emitted by a Bat.
A bat emits an ultrasound of frequency \(50,000\, \text{Hz}\) which bounces off a wall and returns to the bat in \(1\, \text{ms}\text{.}\) (a) Ignoring the speed of the bat, how far away is the bat from the wall? (b) What is the wavelength of the ultrasound wave. Use density of air \(1.3\, \text{kg/m}^3\) and bulk modulus \(1 \times 10^5\, \text{Pa}\text{.}\)
Solution.a
From the bulk modulus \(B\) and the density \(\rho\) the speed \(v\) of the speed,
