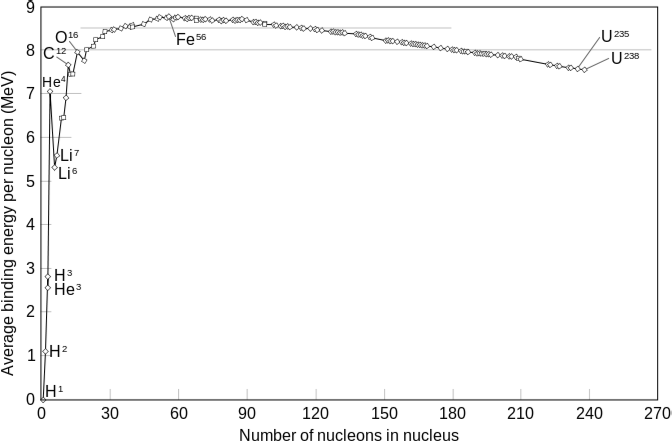
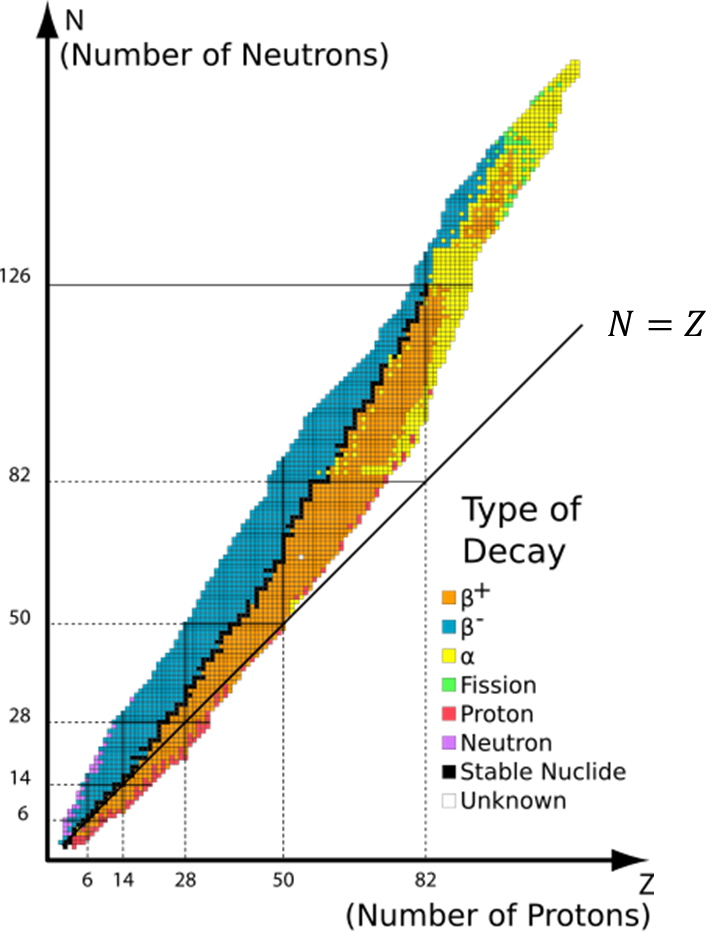The proton has a charge equal to the charge of an electron but of opposite type. The electronic charge has been determined to a very high precision. The following value was posted at the NIST website in April 2014,
\begin{equation*}
e = 1.602 176 565 \times 10^{-19}\:\textrm{C}.
\end{equation*}
with uncertainty in the last two digits. The neutron is electrically neutral. The rest mass of a neutron, \(m_n\text{,}\) is a little larger than the rest mass of a proton, \(m_p\text{.}\) Both are about 1840 times more massive than an electron.
\begin{align*}
\amp m_e =9.109 382 91 \times 10^{-31} \: \textrm{kg}\\
\amp m_p = 1.672 621 777 \times 10^{-27}\: \textrm{kg} = 1836\times m_e\\
\amp m_n = 1.674 927 351 \times 10^{-27}\: \textrm{kg} = 1839\times m_e
\end{align*}
The mass is often quoted in unified atomic mass unit (amu) denoted by the letter u. The quantity u is defined by setting the mass of the isotope \(^{12}_{\ 6}\)C to exactly 12u. This gives the following conversion between u and kg.
\begin{equation*}
1\: \textrm{u} = 1.660 540\times 10^{-27}\:\textrm{kg}.
\end{equation*}
In terms of the unit u the masses of protons and neutrons are
\begin{align*}
\amp m_p = 1.007 276 466 812 \: \textrm{u}\\
\amp m_n = 1.008 664 916 00 \: \textrm{u}
\end{align*}
Recall that the rest mass energy of a particle of rest mass \(m_0\) is given by
\begin{equation*}
E_{\textrm{0}} = m_0 c^2
\end{equation*}
Therefore, the rest mass energies of proton and neutron are
\begin{align*}
\amp E_{0,p} = 1.672 621 777 \times 10^{-27}\: \textrm{kg} \times (3\times 10^{8}\:\textrm{m/s})^2 = 1.50536\times10^{-10} \:\textrm{J}\\
\amp E_{0,n} = 1.674 927 351 \times 10^{-27}\: \textrm{kg}\times (3\times 10^{8}\:\textrm{m/s})^2 = 1.50743\times10^{-10}:\textrm{J}
\end{align*}
These energies are often written in units of MeV or GeV, which stand for million electron volts and giga or billion electron volts.
\begin{align*}
\amp E_{0,p} = 938.272 046 \:\textrm{MeV} =0.938 272 046\:\textrm{GeV} \\
\amp E_{0,n} = 939.565 379 \:\textrm{MeV} = 0.939 565 379\:\textrm{GeV}
\end{align*}
If we divide these rest energies by \(c^2\) then we will get the mass back.
\begin{align*}
\amp m_p = \frac{E_{0,p}}{c^2} = 938.272 046 \:\left[\frac{\textrm{MeV}}{c^2} \right]= 0.938 272 046\:\left[\frac{\textrm{GeV}}{c^2} \right] \\
\amp m_n = \frac{E_{0,p}}{c^2} =939.565 379 \:\left[\frac{\textrm{MeV}}{c^2} \right] =0.939 565 379\:\left[\frac{\textrm{GeV}}{c^2} \right]
\end{align*}
The units \(\textrm{MeV}/c^2\) and \(\textrm{GeV}/c^2\) are commonly used units of mass in nuclear physics. The conversion between u and \(\textrm{MeV}/c^2\) is
\begin{equation*}
1\: \textrm{u} = 931.494 \left[\frac{\textrm{MeV}}{c^2} \right].
\end{equation*}
The estimate of the radius of an atomic nucleus was first obtained by Rutherford and his assistants in their experiments with \(\alpha\)-particles on Aluminum targets. He had deduced a formula of scattering of alpha particles assuming purely Coulombic repulsion between the positively charged alpha particles and the positively charge nucleus. The breakdown of the Rutherford scattering formula suggested a closest approach of the alpha particle to the nucleus, which took to be a measure of the radius of the nucleus. Let \(d_{\textrm{min}}\) be the closest approach of the alpha particles (charge \(+2e\)) to a nucleus (charge \(Ze\)), then equating the kinetic energy of the alpha particle to the potential energy at the closest approach give the following equation.
\begin{equation*}
\frac{1}{4\pi\epsilon_0} \frac{(2e)(Ze)}{d_{\textrm{min}}} = \frac{1}{2} m_{\alpha} v_{\alpha}^2 = K_{\alpha}.
\end{equation*}
Now, if we assume that \(d_{\textrm{min}}\) to be a rough measure of the radius \(R\) of the nucleus we can set \(d_{\textrm{min}} = R\) and obtain the following for \(R\text{.}\)
\begin{equation*}
R = \frac{1}{4\pi\epsilon_0} \frac{2Ze^2}{K_{\alpha}} .
\end{equation*}
Rutherford found that the energy of alpha particles available to him were not sufficient to penetrate the gold nuclei. Facing this challenge, Rutherford is said to have made the famous remark:
“There is no money for apparatus. We shall have to use our heads!”
Rutherford correctly noted that lighter nuclei will be penetrated by the alpha particles from radium source. He directed his students to try Aluminum target and sure enough, they found considerable back scatter. Using \(Z= 13\) and \(K_\alpha = 7.7\) MeV we obtain an estimate of the radius of Aluminum nucleus to be
\begin{equation*}
R_{\textrm{Al}} = [9\times 10^{9}]\times\frac{2\times 13\times (1.6\times 10^{-19})^2}{ 7\times 10^6\times 1.6\times 10^{-19}} = 5.0\times 10^{-15}\:\textrm{m}.
\end{equation*}
The nuclear radius is in the range of tens of femtometers. One femtometer is also referred to as 1 fermi.
\begin{equation*}
1\:\textrm{fermi} = 1\: \textrm{fm} = 1.0\times 10^{-15}\:\textrm{m}.
\end{equation*}
Electron scattering experiments give a better value for the radius of nuclei. The density \(\rho\) of nuclei varies very little among nuclei with the value being
\begin{equation*}
\rho = 2.3\times 10^{17}\:\textrm{kg/m}^3,
\end{equation*}
which is 14-orders of magnitude more than the density of ordinary matter. Neutron stars are supposed to be this dense. A typical neutron star packs in a 12-13 km ball a mass equal to 1.4-3.2 solar masses with one solar mass being \(\sim 2\times 10^{30}\) kg. For a nucleus, the mass will come from the nucleons. Let \(m\) be mass per nucleon, \(A\) be the number of nucleon, and \(R\) be the radius of a nucleus, then we can write the following equation for the total mass.
\begin{equation*}
m \times A = \rho \times \frac{4}{3} \pi R^3.
\end{equation*}
This gives the following useful relation.
\begin{equation}
R = R_0 A^{1/3},\tag{54.1}
\end{equation}
with
\begin{equation*}
R_0 = \left[ \frac{3m}{4\pi\rho}\right]^{1/3}
\end{equation*}
Using \(m = 1\) u for the average mass of the nucleon, we fin the value of \(R_0\) to be
\begin{equation*}
R_0 = 1.2\times 10^{-15}\:\textrm{m}.
\end{equation*}
The relation in Eq.
(54.1) is direct consequence of the constancy of the nuclear density.