Skip to main content

Section 48.2 The Human Eye

The human eye is perhaps the most important optical instrument. In many instruments human eye plays an important role in observing the image. Therefore, a good understanding of optics of the human eye is very useful.

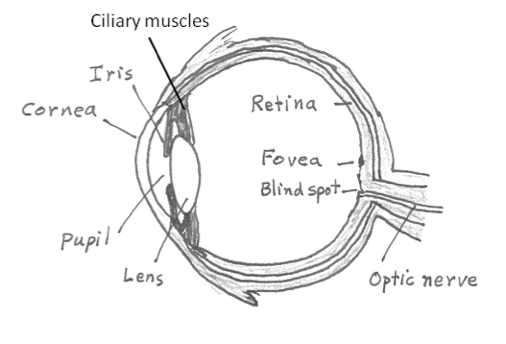
Figure 48.2.1 shows a rough sketch of the human eye. You can liken the eye to a camera. Light enters the eye through the pupil, which is the black hole at the centre of the iris. The size of the pupil is controlled by the iris. The pupil appears black because hardly any light is reflected from it.

Light then passes through a converging lens system consisting of the cornea and the eye lens. The light then is focused on the retina which is a sensitive detector of light. The retina consists of light-sensitive cells, called rods and cones, and nerve endings. The retina converts light energy into electrical signals, which are then carried to the visual cortex of the brain for further processing, which gives rise to the sense of vision.

Figure 48.2.1. Schematic drawing of a human eye.

Subsection 48.2.1 Accomodation and Near Point

Contrary to the popular belief, most of the bending of light rays in the eye is not done by the eye lens but by the cornea, which is itself a converging lens of focal length approximately \(2.3\text{ cm}\text{.}\) A finer focusing is done by the eye lens, a converging lens of less power than cornea, having a focal length of approximately 6.4 cm. The ciliary muscles adjust the shape of the eye lens for focusing on the nearby and far objects. By changing the shape of the eye lens the eye can change the focal length of the lens. This mechanism of the eye is called the accomodation. When the ciliary muscles are relaxed the lens is thin and has a longer focal length so that the distant objects are properly focused at the retina. For a nearby object, the eye needs a smaller focal length lens, which is achieved by contracting the ciliary muscles and thereby thickening the lens.

The sharpness of the image seen by the eye also depends on the distance from the eye and whether the eye muscles are relaxed or contracted. The nearest point an object can be placed so that eye can form a clear image on the retina by maximum accommodation is called the near point of the eye.

To find the sharpest image point for you, hold a sharpened pencil at an arms length and look at its tip with your naked eye. Now, as you bring the pin closer, you should notice that there is point at which the tip of the pencil appears clearest. That would be your near point. For most people the near point is around 25 cm from the eye. For the best view you want whatever you are looking at to be at the near point. Similarly, there is a far point, which is the farthest distance an object is clearly visible.

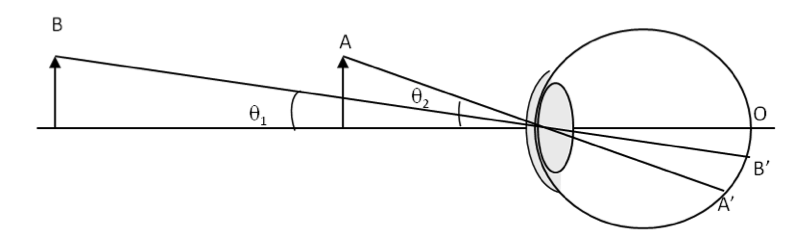
The apparent size of the object perceived by the eye depends on the angle the object subtends on the eye. The same object appears smaller when it is moved farther away. As shown in Figure 48.2.2 when the object is at A, it subtends a larger angle at the eye and hence forms a larger image \(\text{OA}^{\prime}\text{,}\) than when it is moved farther away to B. Since the near point of your eye is the closest distance for clear image you would perceive an object to be the largest and clearest if it is at the near point.

Figure 48.2.2. Size determined by an eye by angle subtended by it.

Subsection 48.2.2 Common Eye Defects

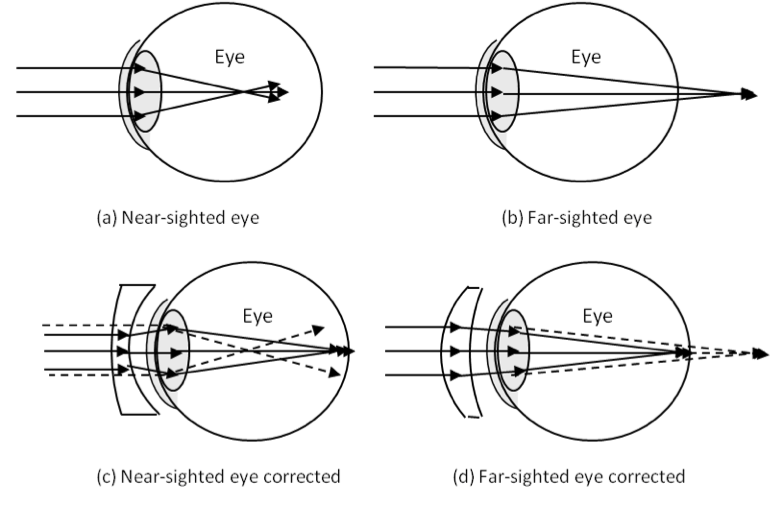
The most common types of eye defects are near-sightedness and far-sightedness in which rays coming into the eye do not focus on the retina without assistance of an external lens as illustrated in Figure 48.2.3. In a near-sighted eye parallel rays focus in front of the retina. That is, in the near-sighted eye the focal length of the lens is shorter than required to focus on the retina. A concave lens in front of the eye bends the rays outward and helps focuses them on the retina. The far-sighted eye has the opposite problem with parallel rays focusing behind the retina. To correct for this one needs a converging lens in front of the eye.

Figure 48.2.3. Common eye problems related to focusing.

The focal length of corrective lenses is specified by giving its optical power in diopters, abbreviated as \(\text{D}\) or \(text{dpt}\text{.}\) Optical power is also simply referred to as power. The numerical value of the power of a lens in diopters is the inverse of the focal length expressed in meters.

\begin{equation} P(\text{diopters}) = \frac{1}{f(\text{in meters})}.\tag{48.2.1} \end{equation}

Thus, a prescription of \(-2.5\text{ D}\) would require a lens of focal length \(\frac{1}{2.5}\text{ m}\text{,}\) i.e., \(4\text{ cm}\text{.}\) The negative sign means that you need a diverging lens.

Subsection 48.2.3 Optical Power of Eye

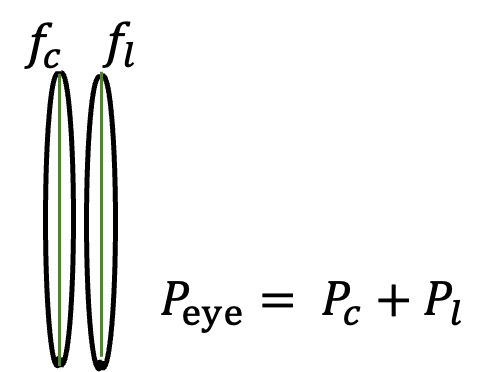
We take a digression here to remind the student that when you stack lenses together, then the entire set of lenses can be treated as one lens of power equal to the sum of the powers of individual lenses. In the eye, there are two converging lenses, the cornea and the eyelens. The net power is the sum of the power of the two as illustrated in Figure 48.2.4.

Suppose \(f_c\) be the focal length of cornea and \(f_l\) the focal length of the eyelens. Then, effective power of eye will be

\begin{equation*} P_\text{eye} = P_c + P_l. \end{equation*}

Writing this in terms of focal length of eye as one converging lens, not two - the cornea and eyelens, will be

\begin{equation} \frac{1}{f_\text{eye}} = \frac{1}{f_c} + \frac{1}{f_l}.\tag{48.2.2} \end{equation}

Figure 48.2.4. Common eye problems related to focusing.

Subsection 48.2.4 Eye as an Optical Instrument

We can think of eye as an optical instrument with pupil serving as the aperture stop. The diameter \(D_\text{pupil}\) of the pupil will be the aperture diameter of the instrument and effective focal length \(f_\text{eye}\) the focal length of the instrument. Therefore, we can write the F/# of the eye to be

\begin{equation*} F/\# = \frac{f_\text{eye}}{D_\text{pupil}}. \end{equation*}

Using typical numbers for the eye, say, \(f_\text{eye} = 2.0\ \text{cm}\text{,}\) and \(D_\text{pupil}= 5\text{ mm}\text{,}\) the F/# of a typical eye will be

\begin{equation*} F/\# = \frac{20\text{ mm}}{ 5\text{ mm}} = 4. \end{equation*}

This would place eye in relatively “fast” instrument category. Note that pupil size varies - its larger when you are in dark for a while and gets smaller wwhen in bright. It might range from about \(2\text{ mm}\) to about \(8\text{ mm}\text{.}\)

You may wonder about the magnification of an eye. Magnification of an unaided eye is 1, i.e., no magnification. You can check this in the formula of magnification of a magnifying glass by setting \(f=\infty\) since that would be a lens with flat ends.

\begin{equation*} M_\text{eye} = 1.0 \end{equation*}

The cornea and eye lens have the focal lengths \(2.3\text{ cm}\) and \(6.4\text{ cm}\) respectively. Find the net focal length and power of the eye.

Hint

Use the sum of powers.

Answer

\(f_\text{eye} = 1.78\ \text{cm}\text{,}\) \(P_\text{eye} =59\ \text{D}\text{.}\)

Solution

The focal length of a combination of lenses add in inverses. Therefore

\begin{equation*} \frac{1}{f_\text{eye} } = \frac{1}{f_{\text{cornea}}} + \frac{1}{f_{\text{lens}}} = \frac{1}{2.3\ \text{cm}} + \frac{1}{6.4\ \text{cm}} \end{equation*}

Hence, the focal length of eye (cornea and lens together)

\begin{equation*} f_\text{eye} = 1.78\ \text{cm}. \end{equation*}

To get the power in \(\text{D}\text{,}\) we first change the unit to \(\text{m}\) and the invert it.

\begin{equation*} P_\text{eye} = \frac{1}{0.0178\ \text{m}} = 59\ \text{D}. \end{equation*}

The net focal length of an eye is \(1.7\text{ cm}\text{.}\) An object is placed at the near point of \(25\text{ cm}\text{.}\) How far behind the lens a focused image is formed? What is the orientation of the image reltive to that of the object?

Hint

Use the lens equation.

Answer

\(1.8\ \textrm{cm} \text{,}\) inverted.

Solution

This is a simple lens problem with object distance given to be \(p = 25\text{ cm}\text{.}\) Therefore, we determine the image distance from the lens equation.

\begin{equation*} \frac{1}{q} = \frac{1}{f} - \frac{1}{p} = \frac{1}{1.7\ \textrm{cm}} - \frac{1}{25\ \textrm{cm}} \end{equation*}

Hence,

\begin{equation*} q = 1.8\ \textrm{cm}. \end{equation*}

Therefore the image is formed \(1.8\text{ cm}\) behind the lens.

To find the orientation, we look at the sign of magnification, which, in terms of \(p\) and \(q\) is

\begin{equation*} m = - \frac{q}{p} = - \frac{1.8\ \textrm{cm}}{25\ \textrm{cm}} \lt 0. \end{equation*}

Since \(m\lt 0\text{,}\) the image would be inverted in orientation with respect to the object.

A near-sighted eye cannot focus on a far object without a corrective lens. Consider a near-sighted eye that has a far point of \(52\text{ cm}\text{,}\) i.e. when the eye is relaxed the furthest point the person can see clearly is \(52\text{ cm}\) from the eye. Find the power of the corrective lens in diopters needed if the lens is places \(2\text{ cm}\) from the eye.

Hint

There are two physical situations here. In one situation, you don't have any corrective lens and in the other you have a two-lens problem.

Answer

\(-2.0\text{ D}\text{.}\)

Solution

Here we have two physical situations, one without the corrective lens and the other with a diverging corrective lens. The common aspect of the two situations is the image distance of the image formed by the eye. We will generate equations in the two situations and equate \(q_\text{eye}\) from both. That should give us the condition for detemining the focal length of the corrective lens.

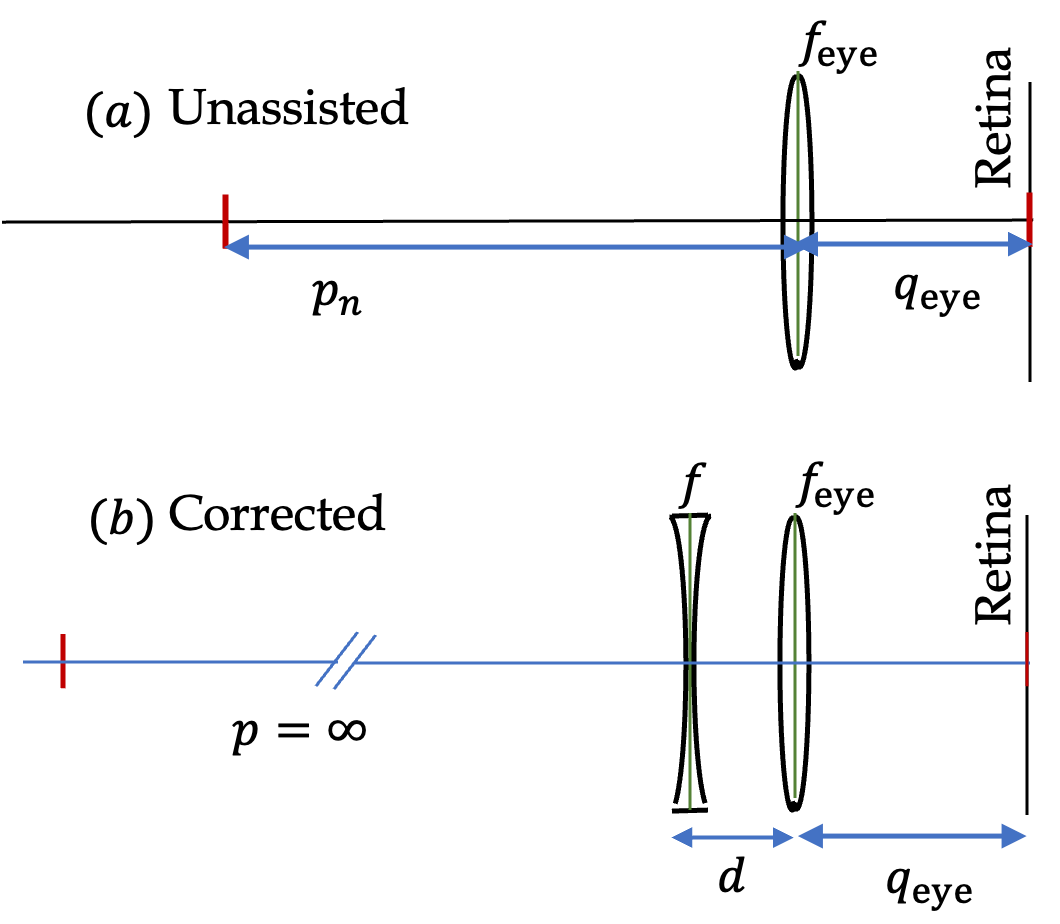
Figure 48.2.8. Figures for Checkpoint 48.2.7

Figure 48.2.8 shows the two situations. Working with the (a), we arrive at

\begin{equation} \frac{1}{q_\text{eye}} = \frac{1}{f_\text{eye}} + \frac{1}{p_n}, \label{eq-exercise-human-eye-near-sighted-unassisted}\tag{48.2.3} \end{equation}

where \(p_n=52\text{ cm}\text{,}\) the near point given in the problem statement. There are two unknowns here \(q_\text{eye}\) and \(f_\text{eye} \text{.}\) We will set up second equation from the second situation.

Now, we work with (b) of the figure. Here, a point at infinity is to be focussed on the retina. Let us use symbol \(f\) for the focal length of the corrective lens, which we seek here. Note that this will be negative. First, the corrective lens, will have image at its first focal point since

\begin{equation} \frac{1}{\infty} + \frac{1}{q} = \frac{1}{f}\ \ \rightarrow\ \ q = f.\label{eq-exercise-human-eye-near-sighted-corrected}\tag{48.2.4} \end{equation}

This image will be object for eye with the image length \(q_\text{eye}\text{.}\) The object distance for eye will be absolute value of \(q\) and the separation \(d\text{.}\) Therefore, we will have

\begin{equation*} \frac{1}{|q| + d} + \frac{1}{q_\text{eye}} = \frac{1}{f_\text{eye}}. \end{equation*}

Using Eq. (48.2.3), we can get rid of both \(q_\text{eye}\) and \(f_\text{eye}\text{.}\) Then, using \(q=f\) from Eq. (48.2.4), we get

\begin{equation*} \frac{1}{|f| + d} = \frac{1}{p_n}. \end{equation*}

Therefore,

\begin{equation*} |f| = p_n - d = 52-2 = 50\text{ cm}. \end{equation*}

Since it is diverging lens, we have

\begin{equation*} f = -50\text{ cm} = -0.50\text{ m}. \end{equation*}

Therefore, the power of the lens needed is

\begin{equation*} P = \frac{1}{f} = -2.0\text{ D}. \end{equation*}

Another Way: With glasses on, we want an object at \(p=\infty\) to form a virtual image at the far point from the eye, which will be \(52-2=50\text{ cm}\) from the lens. Since it is a virtual image

\begin{equation*} q_\text{lens} = -50\text{ cm}. \end{equation*}

With \(p=\infty\text{,}\) this means

\begin{equation*} f_\text{lens} = -50\text{ cm}. \end{equation*}

Therefore, power of the lens

\begin{equation*} P_\text{lens} = -2.0\text{ D}. \end{equation*}

Most people become far-sighted with age and have difficulty reading. Consider a far-sighted person whose near-point is \(80\text{ cm}\text{.}\) What is the power of the corrective lens needed for reading a magazine placed at a distance of \(20\text{ cm}\) from the lens? Assume, the corrective lens is placed \(2\text{ cm}\) from the eye.

Hint

Answer

Solution

The lens needed should form a virtual image of the print \(80\text{ cm}\) from the eye when the print is placed \(20\text{ cm}\) from the lens. Hence, the focal length of the lens needed is obtained by the thin lens formula as follows.

\begin{equation*} \frac{1}{f} = \frac{1}{p} + \frac{1}{q} = \frac{1}{20\ \textrm{cm}} + \frac{1}{-80\ \textrm{cm}} = \frac{3}{80\ \textrm{cm}} \end{equation*}

Therefore

\begin{equation*} f = \frac{80}{3}\ \textrm{cm}. \end{equation*}

Since, the focal length is positive, we need a converging lens. The power of the lens in diopter is obtained by expressing the focal length in meters and taking the inverse of that.

\begin{equation*} P = \frac{1}{f} = 3.75\ \textrm{D}. \end{equation*}