Section 32.2 Charged Metal
Subsection 32.2.1 Conduction Electrons
Metals are good conductors of electricity. You need quantum mechanics to understand this behavior fully. We will just give bullet points here. Turns out that, in a metal, the highest energy band states of a metal, which is called conduction band, are only partially filled, leaving many states easily accessible without any jump in energy.
Electrons in the conduction band are called conduction electrons. Only a fraction of electrons in a metal are conduction electrons. For instance, in a piece of sodium, which is a good conductor, only one out of eleven electrons is condution electron. In copper, only one electron in 29 electrons is conduction electron.
Subsection 32.2.2 Polarization of Metal Near an External Charge
When you place a piece of a metal near a positive charge, as shown in Figure 32.4 the conduction electrons in the metal will be attracted to the external positive charge and migrate freely towards that region.
The region the electrons move to now will have an excess of electrons over protons in the nuclei of atoms and the regions from where the electrons have migrated out will have more protons than electrons. Consequently, the metal will develop a negative region near the external positive charge and a positive region at the far end.
This separation of equal magnitude and opposite type is called polarization of the metal body. If you remove the external charge, the electrons migrate back and neutralize the positive region. The polarization of the metal happens only when there are external charges around.
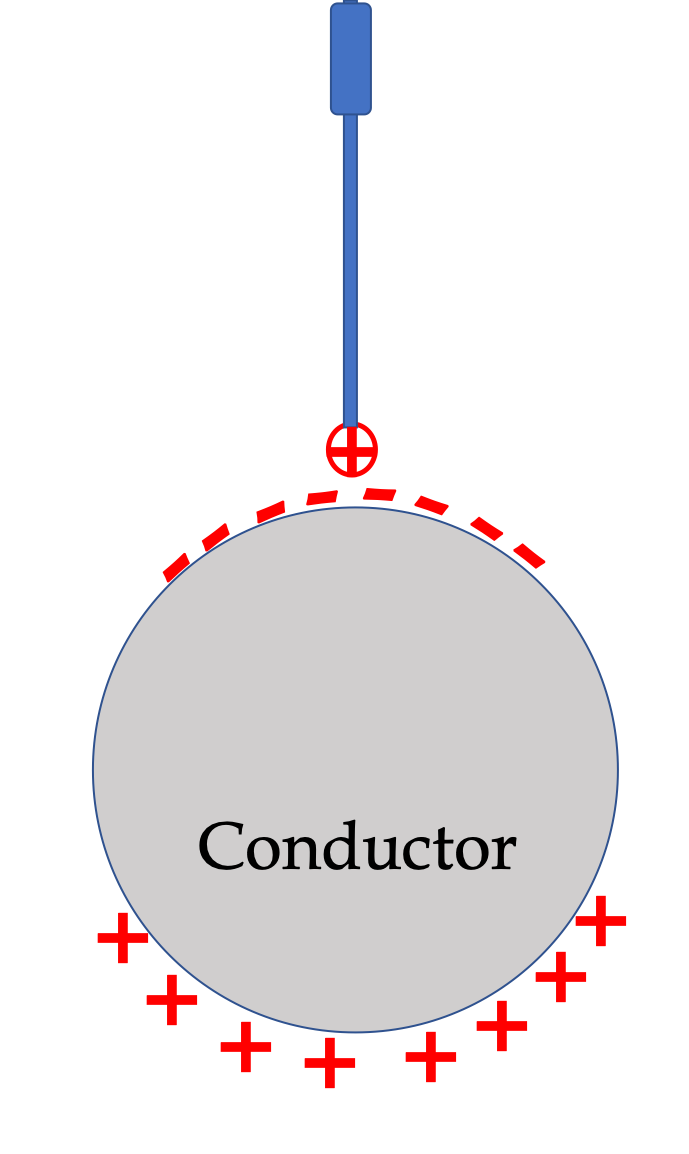
You can think of polarization in terms of electric fields also as shown in Figure 32.5. The external charge creates an electric field, which we can call external electric field. For brevity, only three (blue) E-field lines from external charge are shown in the figure. Drift of conduction electrons leads to the polarization which creates electric field in addition to the external electric field. The external electric field and internal electric field cancel each other inside the metal when conduction electrons in the metal have stopped drifting.
\begin{equation*}
\vec E_\text{net,in metal} = \vec E_{\text{external } q} + \vec E_{q\text{ on metal} } = 0.
\end{equation*}
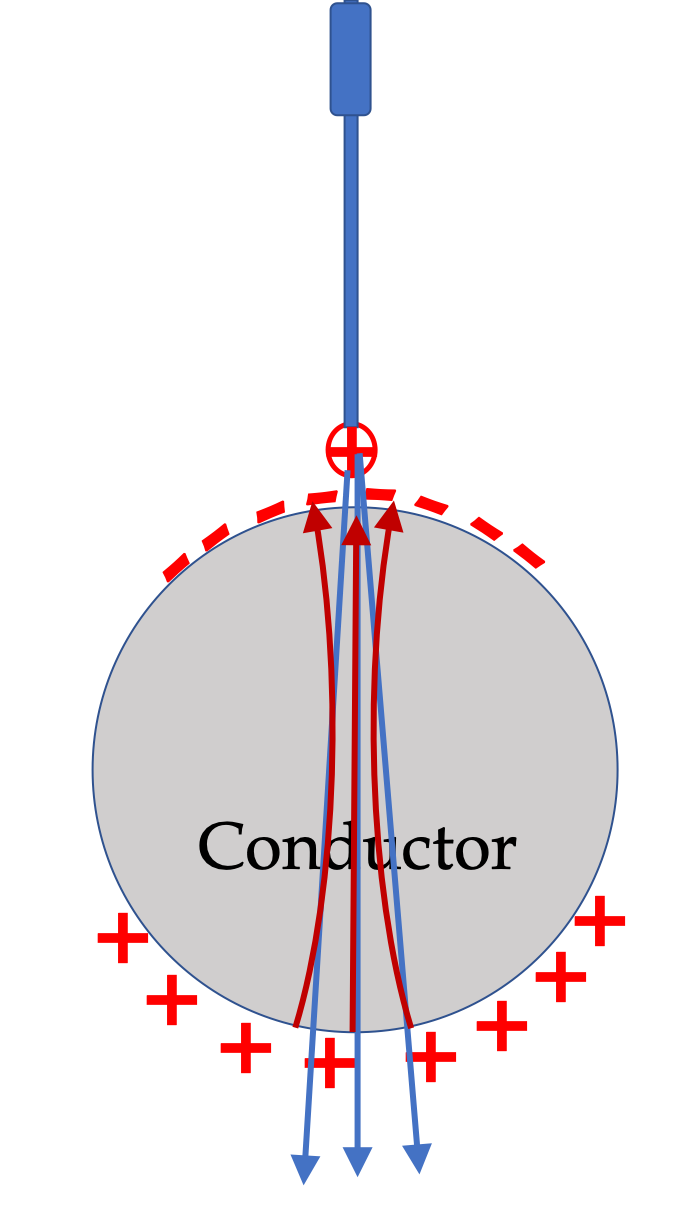
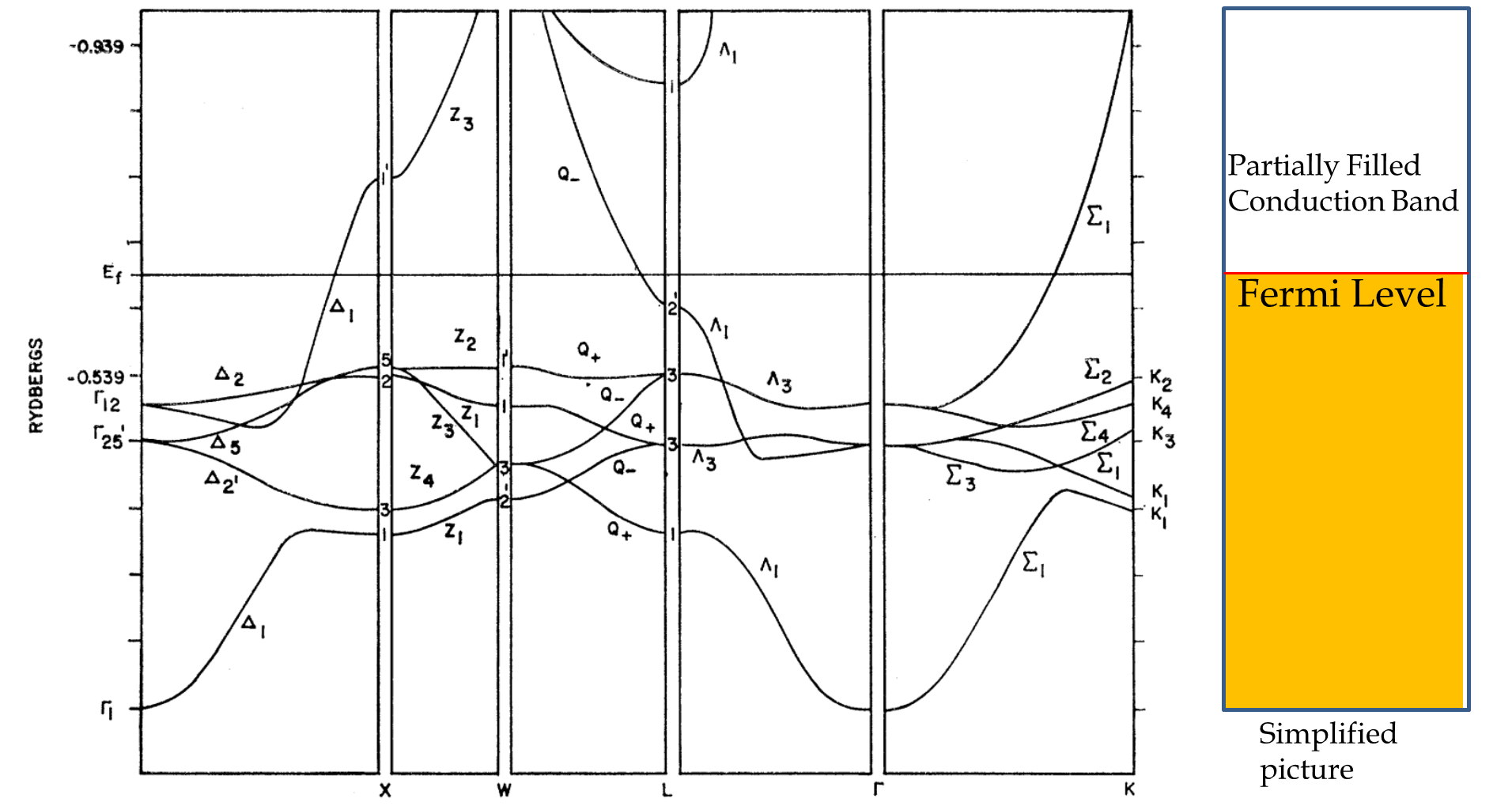
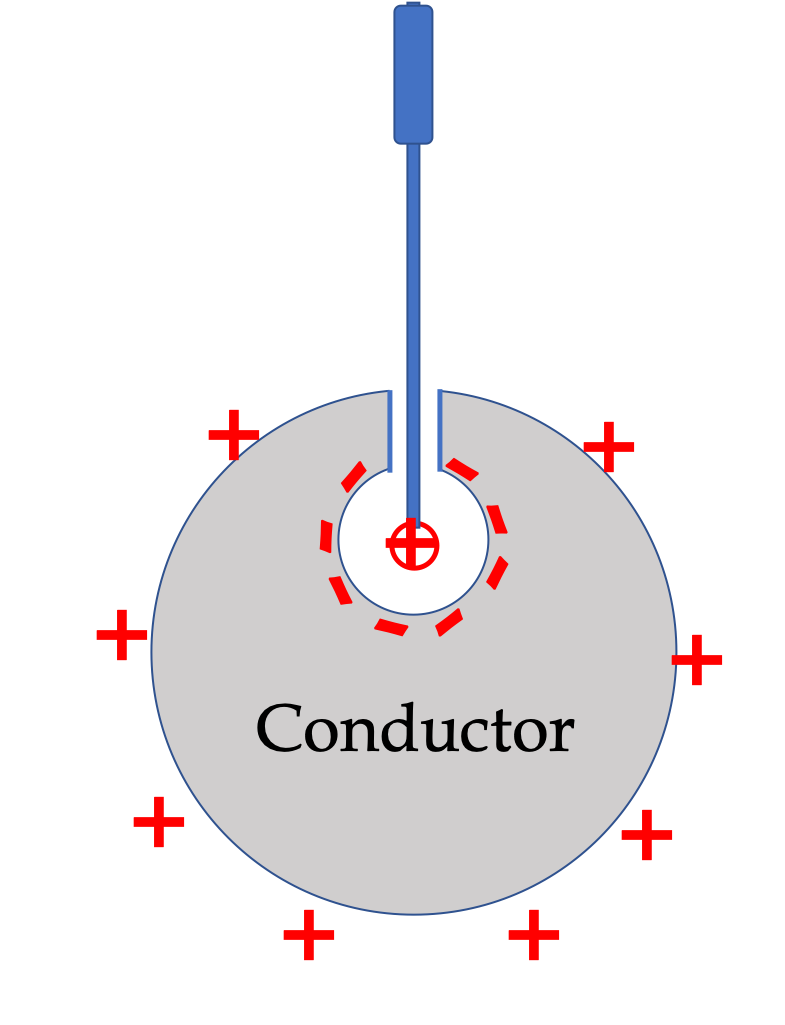
Separation of charges always causes the regions of extra charges on the metal on the outside surface as long as the external charge is outside the metal. This occurs even when there is a cavity in the metal. This is due to the fact that in the static equilibrium, electric field must be zero in the body of the metal. If metal has a cavity and external charge is inside the cavity, the inner surface of the cavity develops extra charge as illustrated next.
If an external charge is placed inside a metal cavity, as shown in Figure 32.7 metal is polarized with opposite charge at the surface of the metal inside the cavity and same charge as the external charge at the outisde surface. The total charge at the inner surface has the same magnitude as the external charge so that zero electric field flux through a surface with all surface points in the metal and surrounding the external charge and charges at the internal surface.
Subsection 32.2.3 Charging a Metal
The inside surface versus the outside surface
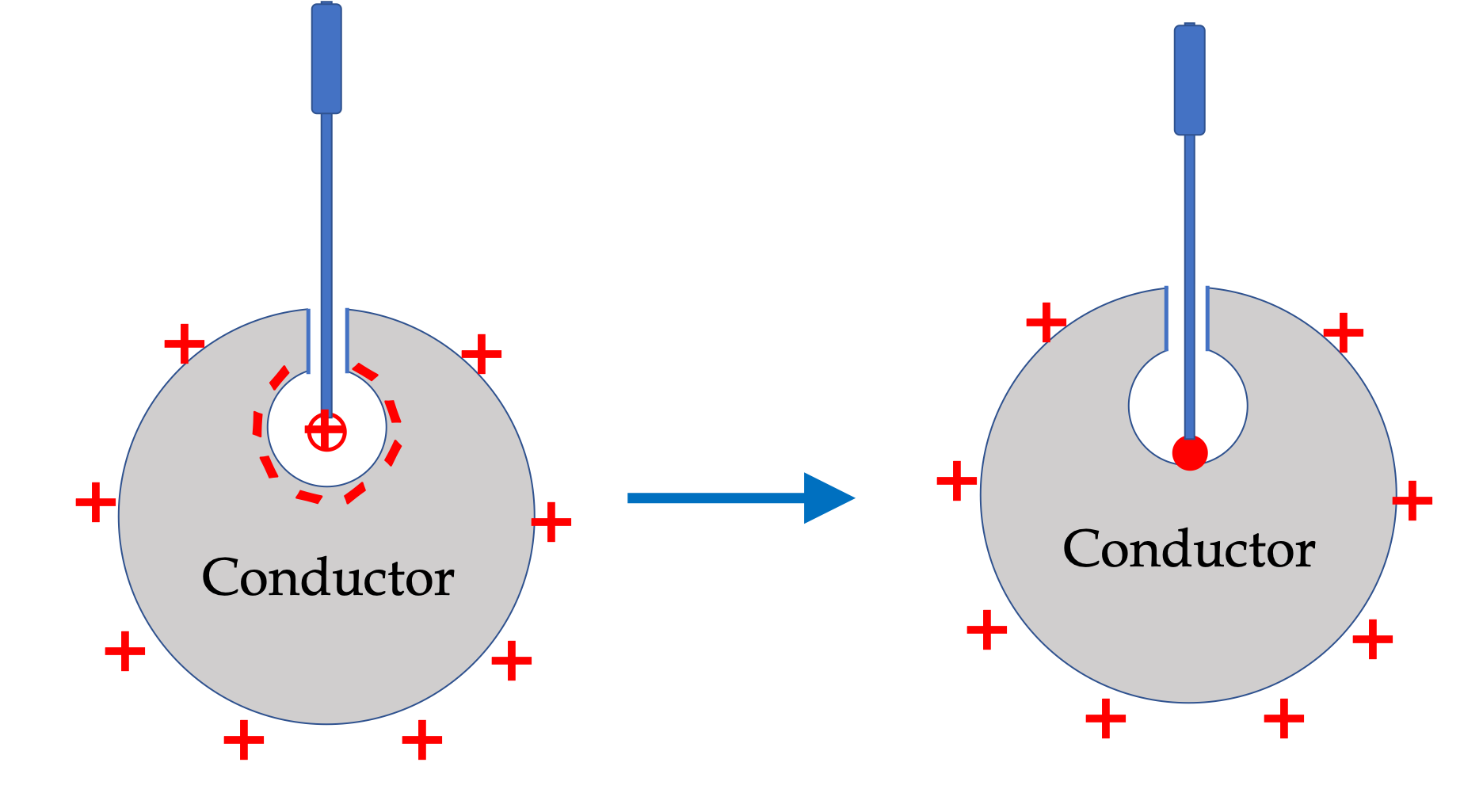
An interesting property of a conductor in a static equilibrium is that extra charges on an isolated conductor end up at the outer surface of the conductor no matter where they originate from.
Consider a situationwhen we bring an external positive charge inside the cavity of a metal and then touch at the inside surface, as shown in Figure 32.8. Before, we touch metal with the external charge, inside surface the cavity is negatively charged and the outside surface of the conductor is positively charged. When we touch the inside surface of the cavity the induced charge is neutralized leaving the outside surface and the whole metal charged with a net positive charge.
Subsection 32.2.4 Distribution of Charges on the Metal Surfaces
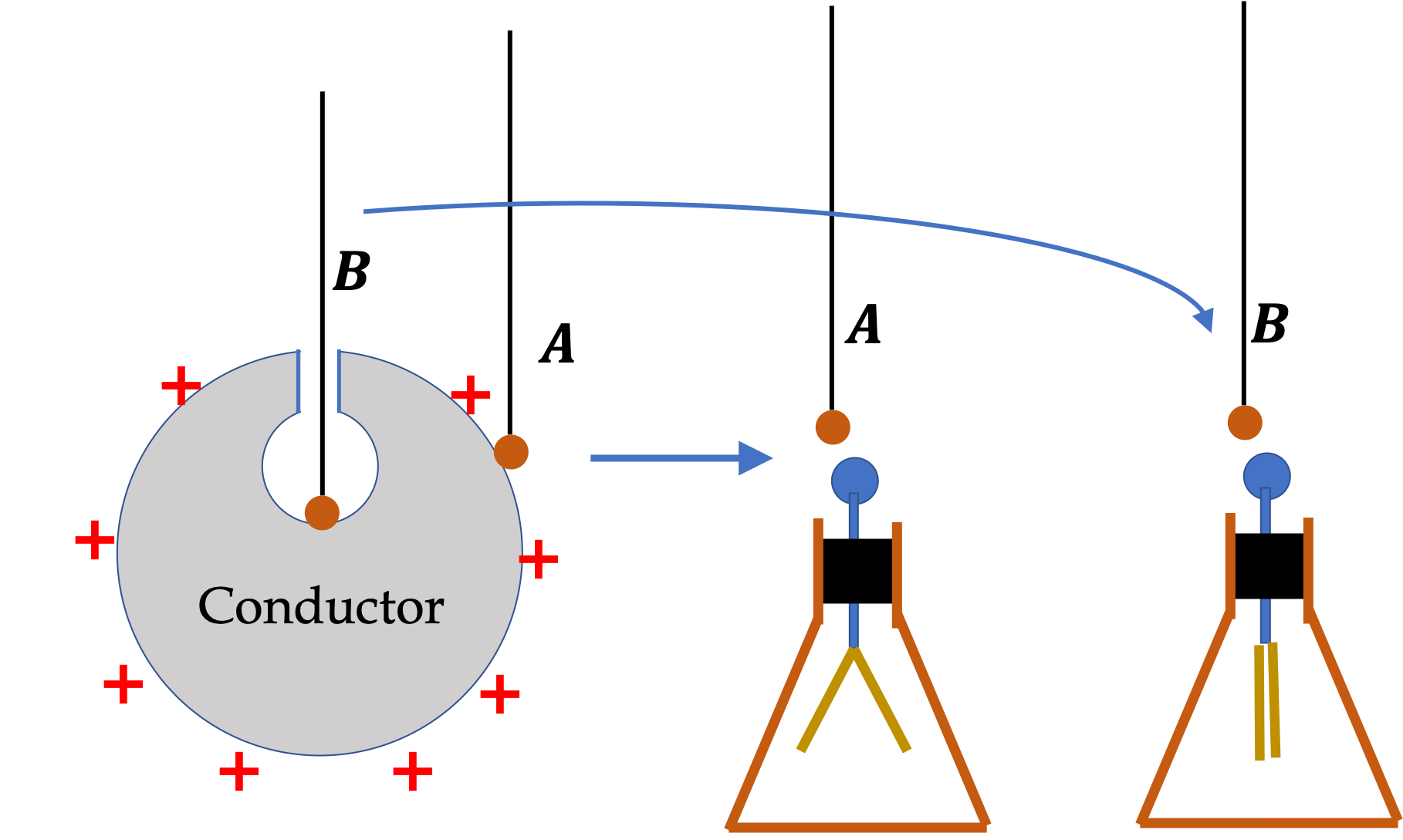
An electroscope is an ingenous device for detecting charges. It is based on two facts (1) charges flow in coductors and (2) like charges repel. In Figure 32.9, we illustrate the use of an electroscope to prove that there are no charges on the inside surface of the cavity.
Bring a small metal ball suspended by an insulating cord, touch the outside surface of the chaged metal, and then bring the ball near the knob of an electroscope. You will find that the leaves of the electroscope separate, proving that there is charge on the outside surface side of metal. Repeat the experiment by touching the metallic ball to the inside surface of the cavity. When you bring the metallic ball to an electroscope, now you will find no deflection, proving no charge on the inside surface.
Another interesting aspect of charge distribution on a conductor is that charges tend to be denser where the curvature of the surface is greater as demonstrated by the charge distribution on an oblong-shaped metal. The surface charge density will be higher near the tip than the other side as shown in Figure 32.10.
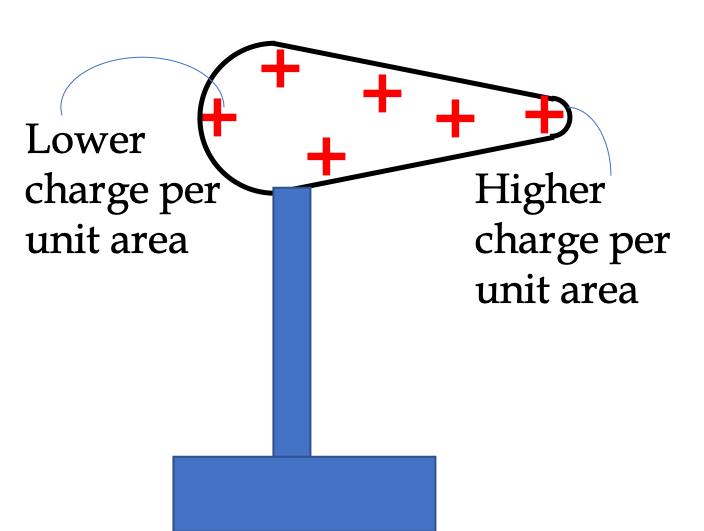
You can use a proof plane, a small flat metal disk with an insulting handle, and an electroscope. Touch different parts of the charged oblong metal with the proof plane, and then touch the knob of an electroscope with the charged proof plane. The amount of deflection of the leaves will be the greatest when the proof plane touches at the tip of the oblong metal, demonstrating a highest charge density at the point when the surface has the largest curvature.

