Water Vapor and Humidity

Section 27.7 Water Vapor and Humidity

The vapor pressure of water plays an important role in atmospheric physics. Humidity\index{Humidity} or dampness of air is a measure of the presence of water molecules in air. Air is mostly made up of the Nitrogen gas (approx. 78% by volume) and the Oxygen gas (approx. 21% by volume). The remaining 1% by volume consists of many other gases, Argon (0.93\%), Carbon dioxide (0.03%), Neon (0.008%), Helium (0.0005%), Hydrogen (0.00005%), varying amounts of water and trace amounts of other gases, where all numbers are given as percentages by volume.

When the temperature of air increases more water is boiled off from oceans and lakes into the vapor phase which changes the ratio of water to other gases in air. Since water molecules are lighter than diatomic nitrogen and oxygen molecules, the density of air decreases when the humidity in the air increases. The humidity or relative humidity of air is defined in terms of partial pressure of water in the air compared to the saturated vapor pressure for the corresponding temperature and pressure.

Partial pressure is a useful concept for mixture of gases. If you go back to the beginning of this chapter and think about how pressure in a gas is related to the motion of molecules, you would find that total pressure of a mixture of gases will be equal to the pressure that each gas will exert if it alone occupied the entire volume at the given temperature. The net pressure of air would be equal to the sum of the partial pressures of the component gases.

\begin{equation*} p_{\text{atm}} = p_{\text{N}_2} +p_{\text{O}_2}+p_{\text{H}_2\text{O}}+\cdots \end{equation*}

Relative humidity is defined as the ratio of partial pressure \(p_{\text{H}_2\text{O}}\) of water actually present in air and the saturated vapor pressure \(p_{\text{H}_2\text{O}}^\text{sat}\) of water expected at that temperature from the coexistence curve of water.

\begin{equation} \text{Relative Humidity} = \frac{p_{\text{H}_2\text{O}}}{p_{\text{H}_2\text{O}}^\text{sat}} \times 100\%.\tag{27.24} \end{equation}

Thus, if the humidity is close to 100% then air holds the maximum amount of water it can under the equilibrium condition. Table 27.20 shows the saturated water vapor pressure and the corresponding densities at various temperatures.

If the relative humidity is more than 100%, then we would have more water vapor pressure than the value of the saturated vapor pressure for that condition. We say that the air is supersaturated with water. When the air is supersaturated with water, water will precipitate out of air. This is what happens at night when temperature drops but water vapor content remains the same as in the evening.

Suppose there is 50% humidity at \(77^{\circ}\text{F}\text{,}\) which corresponds to a partial pressure of 0.5 \(\times\) 23.76 = 11.88 mmHg from Table 27.20 . Now, if the temperature overnight drops to \(50^{\circ}\text{F}\) without any change in partial pressure of water molecules in the air. At \(50^{\circ}\text{F}\text{,}\) saturated vapor pressure is 9.21 mmHg. That means that when the temperature drops to \(50^{\circ}\text{F}\text{,}\) the air will be supersaturated. The water vapor will then condense to liquid. This is the process behind dew formation at night. The dew point is the temperature at which the partial pressure of water in a given atmospheric condition will correspond to the saturated vapor pressure for that condition. In our example of 50% humidity at \(77^{\circ}F\text{,}\) the dew point will be the temperature at which the saturated vapor pressure is \(11.8\) mmH, which is between \(55.4^{\circ}\text{F}\) and \(57.2^{\circ}\text{F}\) as extrapolated from the data in Table 27.20.

Table 27.20. Saturated vapor pressure and saturated vapor densities of water (Ref: http://hyperphysics.phy-astr.gsu.edu/hbase/kinetic/watvap.html\#c1)

Temp	Temp	Saturated	Saturated	Temp	Temp	Saturated	Saturated
(\(^{\circ}\text{C}\))	(\(^{\circ}\text{F}\))	Vapor	Vapor	(\(^{\circ}\text{C}\))	(\(^{\circ}\text{F}\))	Vapor	Vapor
		Pressure	Density			Pressure	Density
		(mmHg)	\((\text{g/m}^3)\)			(mmHg)	\((\text{g/m}^3)\)
-10	14	2.15	2.36	60	140	55.3	51.1
0	32	4.58	4.85	80	176	355.1	293.8
5	41	6.54	6.8	95	203	634	505
10	50	9.21	9.4	98	208	707	560
15	59	12.79	12.83	100	212	760	598
20	68	17.54	17.3	101	214	788	618
25	77	23.76	23	110	230	1074.6	...
30	86	31.8	30.4	120	248	1489	...
40	104	55.3	51.1	200	392	11659	7840

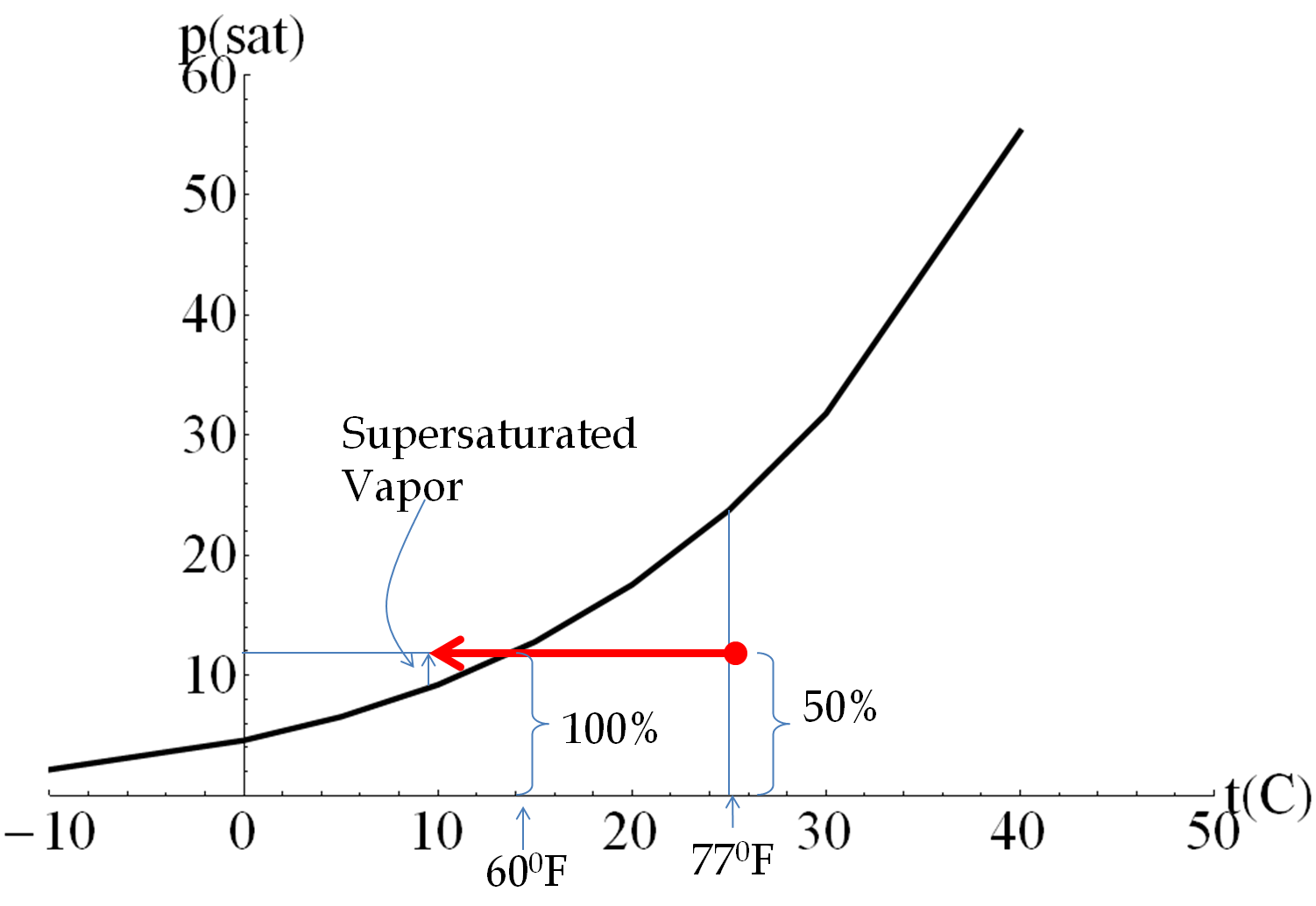
Example 27.21. Partial Pressure of Water Vapor from Humidity.

A meteorologist reports that the humidity is \(60\%\) on a day when temperature is \(77^{\circ}\text{F}\text{.}\) What is the partial pressure of water in the air that day?

Answer.

\(15.689\ \text{mmHg}\text{.}\)

Solution.

We look up the saturated vapor pressure from the table. At \(77^{\circ}\text{F}\) the \(p_\text{sat} = 23.76 \text{ mmHg}\text{.}\) Therefore, \(60\%\) humidity will correspond to the partial pressure \(p\) of water to be

\begin{equation*} 66\% = \frac{p}{23.76\ \text{mmHg}}\ 100\%. \end{equation*}

Solving for \(p\) we obtain

\begin{equation*} p = 15.689\ \text{mmHg}. \end{equation*}

Exercises Exercises

1. Amount of Water to Remove to Reducing Humidity in a Room.

Humidity in a room is to be maintained at \(30\%\text{.}\) On a humid day the humidity is found to be \(80\%\text{.}\) If the room has a volume of 8 cubic meters, how much water vapor must be removed n units of grams?

Data: Saturation pressure, \(p_\text{sat} = 40\ \text{mmHg} \text{.}\)

Hint.

Get partial pressure of water from \(p_{w} = h\, p_\text{sat}\text{,}\) where \(h\) is the humidity as fraction. Then, use ideal gas law.

Answer.

\(300\text{ grams}\text{.}\)

Solution.

Let \(p_\text{sat}\) be the saturation pressure corresponding to the temperature. Then, the partial pressure \(p_{w}\) of water in the room is

\begin{equation*} p_{w} = 0.8 p_\text{sat}. \end{equation*}

Using ideal gas for water molecules filling the volume \(V = 8\text{ m}^3\) space, the number of moles on water in the room would be

\begin{equation*} n = \frac{p_w V}{RT}. \end{equation*}

When the partial pressure is 30\% we would have

\begin{equation*} n' =\frac{p'_w V}{RT} \end{equation*}

The amount to be removed

\begin{equation*} \Delta n = \frac{p_\text{sat} V}{RT} \left(0.8-0.3 \right). \end{equation*}

Using

\begin{equation*} p_\text{sat} \sim 40\ \text{mmHg} =(40 /760)\ \text{atm},\ \ T = 300\ \text{K}, \end{equation*}

the volume in L,

\begin{equation*} V = 8\ \text{m}^3 = 8000\ \text{L}. \end{equation*}

give

\begin{equation*} \Delta n = 17\ \text{mol}, \end{equation*}

which corresponds to about \(300\text{ grams}\) or \(300\text{ cm}^3\) of water.

2. Plotting Saturated Water Vapor Pressure Versus Temperature.

(a) Plot the saturated vapor pressure of water versus the temperature. (b) Plot the saturated vapor density of water versus temperature. (c) On your plots, show what happens when you start with 50\% humidity at \(77^{\circ}\)F and lower the temperature to \(50^{\circ}\)F, and describe in words what you expect.

Solution. a

I will draw only the saturated vapor pressure versus temperature from the table. A similar plot of saturated density versus temperature is left for the student.

Figure 27.22 shows that as you lower the temperature, the state of the atmosphere moves horizontally to the left. At \(77^{\circ}\)F the partial pressure of water is only \(50\%\) of the saturated vapor pressure but when the temperature reaches around \(60^{\circ}\)F, the partial pressure of water is \(100\%\) of the saturated vapor pressure. Lowering the temperature further gets us into the region of super-saturated atmosphere.

Prev Top Next