Section 40.7 Magnetic Materials Bootcamp
Subsection 40.7.1 Magnetic Dipoles
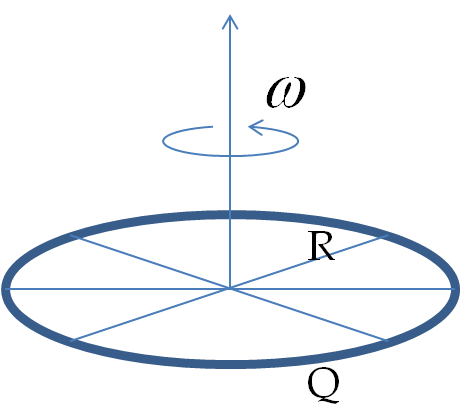
Problem 40.7.1. Magnetic Moment of an Orbitting Electron.
Follow the link: Checkpoint 40.1.5.
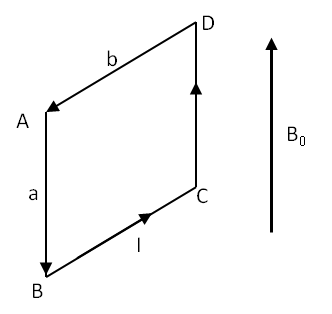
Problem 40.7.2. Rotating a Nuclear Magnetic Dipole in a Magnetic Field.
Follow the link: Checkpoint 40.1.6.
Problem 40.7.3. Torque on a Magnetic Dipole and Energy Required to Flip its Orientation.
Follow the link: Checkpoint 40.1.7.
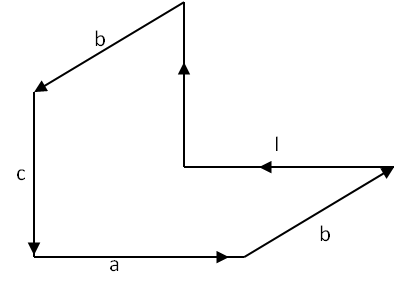
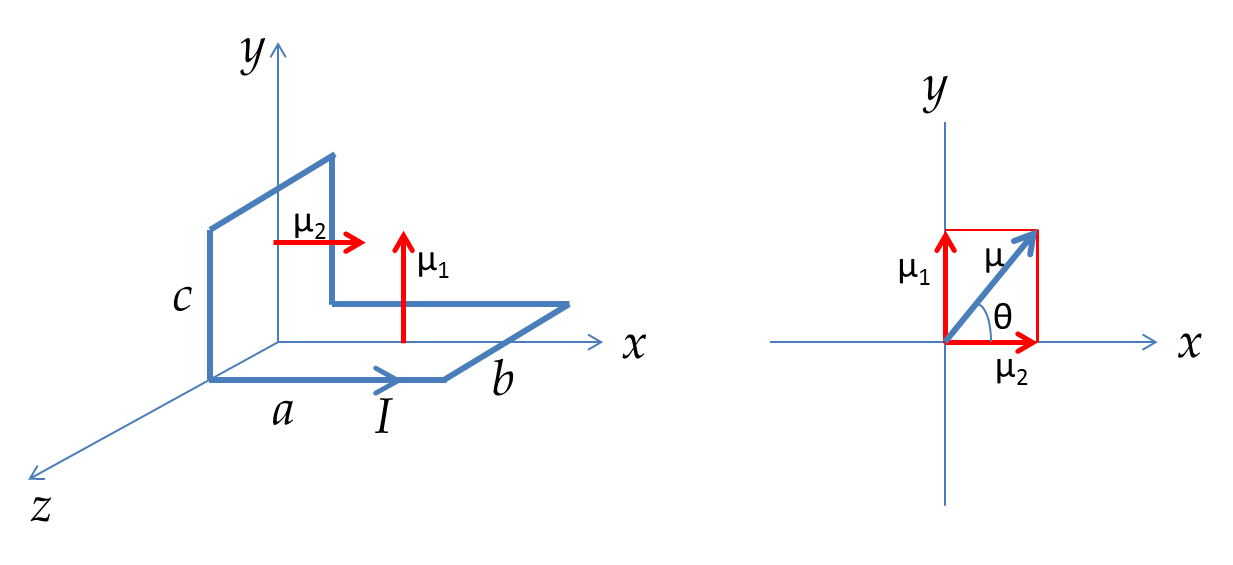
Problem 40.7.4. Magnetic Dipole Moment and Equivalent Circulating Current from Torque.
Follow the link: Checkpoint 40.1.8.
Problem 40.7.5. Force on a Magnetic Dipole in an Nonuniform Magnetic Field of a Bar Magnet.
Follow the link: Checkpoint 40.1.9.
Problem 40.7.6. Energy for Rotating a Magnetic Dipole by an Arbitrary Angle.
Follow the link: Checkpoint 40.1.10.
Problem 40.7.7. (Calculus) Force on a Magnetic Dipole in a Nonuniform Magnetic Field Given in Analytic Form.
Follow the link: Checkpoint 40.1.11.
Problem 40.7.8. Force and Torque on a Magnetic Dipole Placed in a Solenoid.
Follow the link: Checkpoint 40.1.12.
Problem 40.7.9. Energy for Rotating Magnetic Dipole Moment of Proton.
Follow the link: Checkpoint 40.4.1.
Subsection 40.7.2 Microscopic View of Magnetic Materials
Problem 40.7.10. Calculating Magnetic Moments of Atoms.
Follow the link: Checkpoint 40.2.1.
Problem 40.7.11. Magnetic Moment of a Helium Atom in an Electronically Excited State.
Follow the link: Checkpoint 40.2.2.
Subsection 40.7.3 Induced Dipole Moments
Problem 40.7.12. Induced Magnetic Moment in a Helium Atom.
Follow the link: Checkpoint 40.3.2.
Problem 40.7.13. Induced Magnetic Dipole Moment in Bismuth.
Follow the link: Checkpoint 40.3.3.
Subsection 40.7.4 Magnetization and Magnetic Field
Problem 40.7.14. Magnetism in Copper Rod Carrying Current.
Follow the link: Checkpoint 40.6.5.
Problem 40.7.15. Magnetization of Platinum Inside a Solenoid.
Follow the link: Checkpoint 40.6.6.
Problem 40.7.16. Magnetization of Water from Parallel Currents over its Surface.
Follow the link: Checkpoint 40.6.7.
Problem 40.7.17. Magnetization of Oxygen Gas by Current in a Wire.
Follow the link: Checkpoint 40.6.8.