Skip to main content
   
Logo image

Physics Bootcamp

Samuel J. Ling

Section 13.11 Harmonic Oscillator Bootcamp

Exercises Exercises

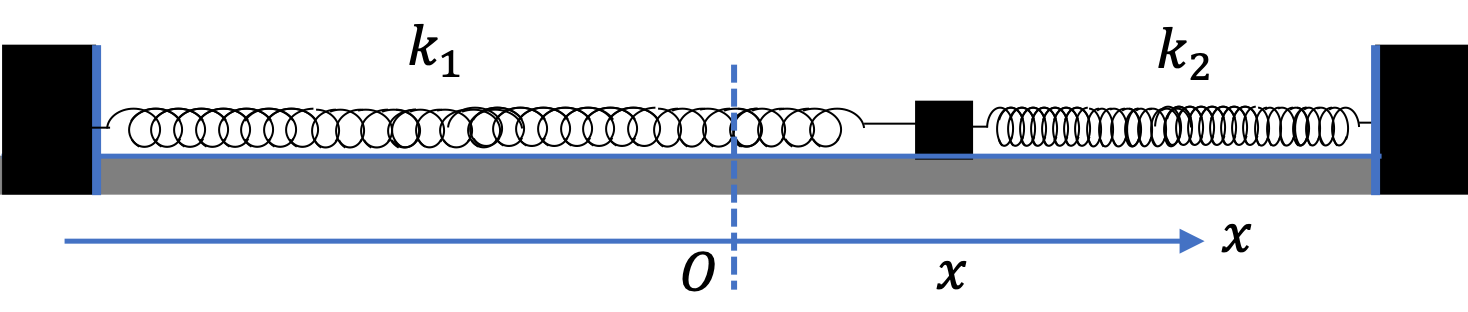
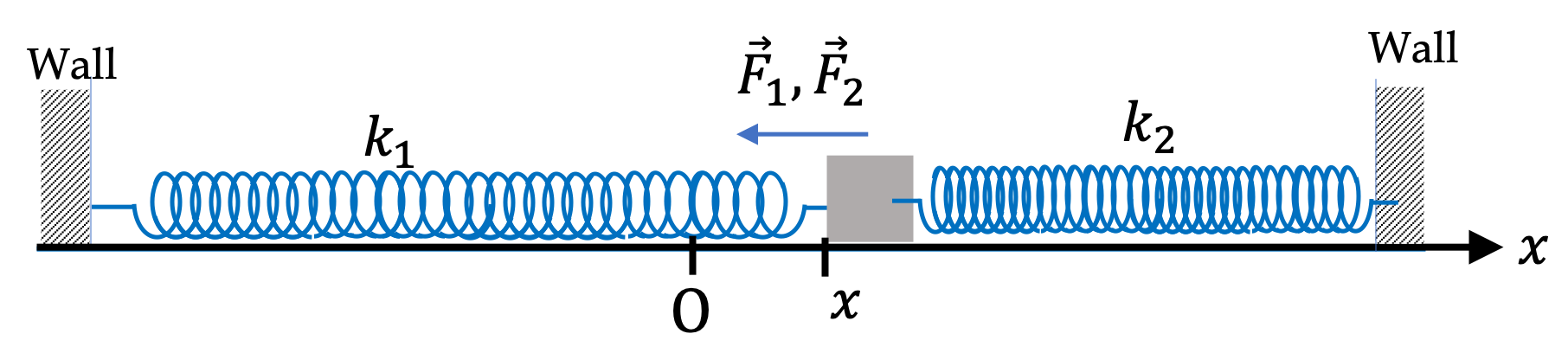
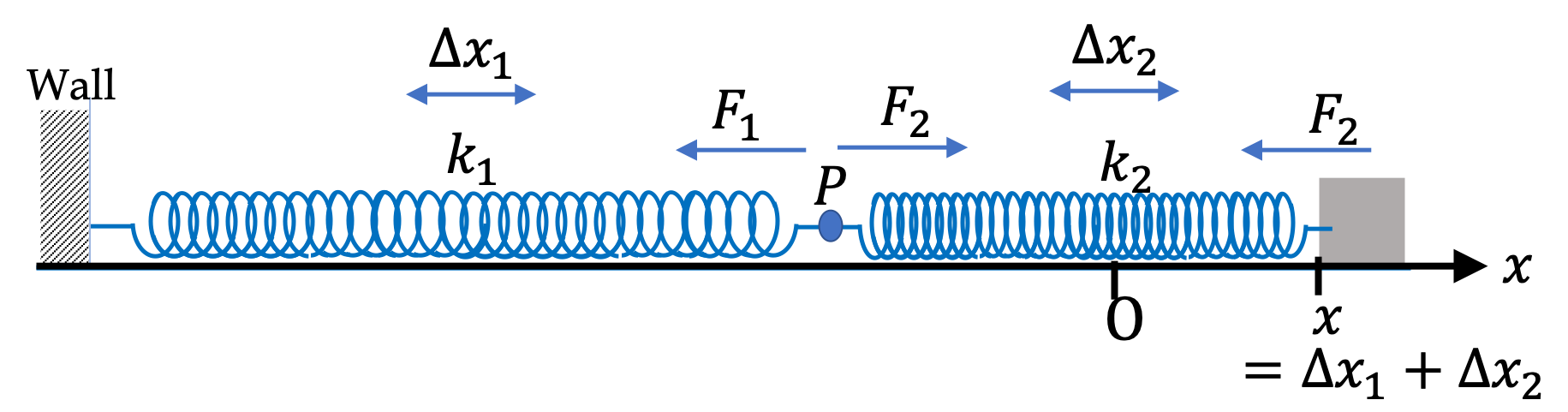
Harmonic Oscillator

1. Mass of an Astronaut from Harmonic Motion.
Follow the link: Example 13.5.
2. Angular Frquesncy, Frequency, and Period of a GHarmonic OScillator.
Follow the link: Exercise 13.2.5.1.
3. Reading Harmonic Oscillator Solutions in Two-Amplitudes Form.
Follow the link: Example 13.10.
4. Reading Harmonic Oscillator Solutions in Amplitude-Phase Form.
Follow the link: Example 13.11.
5. Writing Harmonic Oscillator Solutions in Alternate Forms.
Follow the link: Example 13.12.
6. Harmonic Oscillator Solution for Given Initial Position and Velocity.
Follow the link: Example 13.13.
7. Position Function of an Oscillator from Given Initial Conditions.
Follow the link: Exercise 13.2.5.3.
8. Understanding Positions of Two Oscillators and Their Phasors.
Follow the link: Example 13.14.
9. Comparing Position and Velocity of Two Oscillators with Different Phases.
Follow the link: Exercise 13.2.5.2.
10. Various Forms of the Simple Harmonic Oscillator Solution.
Follow the link: Exercise 13.2.5.5.

Simple Harmonic Systems

11. Simple Harmonic Motion About a Minimum of a Cubic Potential.
Follow the link: Example 13.20.
12. Simple Harmonic Motion About a Minimum of a Quartic Potential.
Follow the link: Exercise 13.3.2.1.

Plane Pendulum

14. Period of Same Pendulum at Different Locations on Earth.
Follow the link: Exercise 13.4.2.2.

Physical Pendulum

15. Frequency of Oscillation of a Swinging Rod.
Follow the link: Exercise 13.5.1.
16. Frequency of Oscillation of a Swinging Rod With a Spherical Ball at End.
Follow the link: Exercise 13.5.2.

Energy of a Harmonic Oscillator

19. Speed of a Block Attached to a Spring.
Follow the link: Example 13.26.
20. Energy of a Simple Harmonic Oscillator at Different Points of the Cycle.
Follow the link: Exercise 13.7.2.1.
21. Analyzing Energy and Motion of a Block Hung from the Ceiling.
Follow the link: Exercise 13.7.2.2.

Damped Harmonic Oscillator

24. Displacement of a Harmonic Oscillator Given Initial Displacement and Velocity.
Follow the link: Exercise 13.8.6.3.
25. Solving a Damped Oscillatory Problem Given Parameters and Initial Conditions.
Follow the link: Exercise 13.8.6.4.

Dissipation of Energy

26. Quality of a Lightly Damped Oscillator.
Follow the link: Example 13.33.
30. Quality Factor from Dynamics of the Displacement of Underdamped Oscillators.
Follow the link: Exercise 13.9.4.3.
31. Energy Dissipation of a Lightly-Damped Oscillator from Solution.
Follow the link: Exercise 13.9.4.4.

Driven Oscillator

34. Phase at Resonance of an Underdamped Driven Oscillator.
Follow the link: Exercise 13.10.8.2.
35. Quality of a Damped Driven Oscillator from Information about Resonance.
Follow the link: Exercise 13.10.8.3.
37. Practice Definitions in Driven Damped Harmonic Oscillator.
Follow the link: Exercise 13.10.8.5.

Miscellaneous

38. Bullet Hit and Embedded in Block Attached to a Spring.
A bullet of mass \(m \) and speed \(v_0 \) is fired horizontally on a block of mass \(M \) attached to a spring of spring constant \(k \) whose other end is fixed to a wall. The direction of the velocity of the bullet is along the length of the spring. Upon impact, the bullet is embedded in the block.
(a) Ignoring damping, find the amplitude of the resulting harmonic motion.
(b) Compare the energy of the bullet before impact to its energy when it momentarily comes to rest after the spring is compressed, i.e., at the turning point of the oscillatory motion. Is the energy of the bullet conserved? Why or why not?
Hint.
(a) Use momentum conservation to find the velocity after impact, then use this to find the amplitude of oscillations, (b) Energy will not be conserved.
Answer.
(a) \(\frac{mv_0}{\sqrt{k(M+m)}}\text{,}\) (b) \(E_i - E_f= \dfrac{M}{ m+M}\, E_i \text{.}\)
Solution 1. (a)
(a) Let \(V\) be the speed of the bullet+block together after the impact. Momentum conservation across the impact gives
\begin{equation*} (m+M) V = mv_0. \end{equation*}
Therefore, immediately after the impact they move at
\begin{equation*} V = \dfrac{m}{m+M}\, v_0. \end{equation*}
The moving combo of block and bullet will compress the spring. Since spring force is a conservative force, we will use conservation of energy to figure out the amount of compression. Let \(x \) denote this quantity when maximally compressed. At that point the masses momentarily come to rest.
\begin{equation*} \dfrac{1}{2}\,k x^2 = \dfrac{1}{2}\, (m+M)\, \left(\dfrac{m}{m+M}\, v_0 \right)^2. \end{equation*}
Simplifying this we get
\begin{equation*} x = \dfrac{mv_0}{\sqrt{k(m+M)}}. \end{equation*}
This is the amplitude of the subsequent oscillations.
Solution 2. (b)
The energy of the bullet before impact is
\begin{equation*} E_{i} = \dfrac{1}{2}mv_0^2. \end{equation*}
At the instant the combo of bullet and block momentarily comes to rest, the bullet has only potential energy
\begin{equation*} E_{f} = \dfrac{1}{2}kx^2 = \dfrac{1}{2}\, \dfrac{m}{ m+M}\, m v_0^2. \end{equation*}
Therefore, the loss in energy of the bullet is
\begin{align*} E_i - E_f \amp = \dfrac{1}{2}mv_0^2 \left( 1 - \dfrac{m}{ m+M} \right), \\ \amp = \dfrac{M}{ m+M}\, E_i. \end{align*}
Energy of the bullet is not conserved since, upon impact, work is done by the block on the bullet.
39. A Block Attached to Two Springs on Opposite Sides.
Two springs of spring constants \(k_1 \) and \(k_2\) are attached on the two opposite sides of a block of mass \(m \) and the free ends of the springs are attached to two fixed supports as shown in Figure 13.45. The block rests on a frictionless flat horizontal surface and can move along the line of the two springs. At equilibrium position of the block, the two springs are neither stretched nor compressed.
When the block is pulled a little, say a distance \(A\text{,}\) from the equilibrium position in the line of the springs and released from rest, the block executes a simple harmonic motion whose frequency depends on the spring constants of the two springs and the mass of the block.
Figure 13.45. Figure for Exercise 13.11.39.
(a) By looking at forces on the block at an arbitrary point in time, find the equation of motion of the block.
(b) From the equation of motion, show that the angular frequency of oscillation is given by \(\omega=\sqrt{\omega_1^2+\omega_2^2}\text{,}\) where \(\omega_1^2 = k_1/m\) and \(\omega_2^2 = k_2/m\text{.}\)
(c) What is the kinetic energy of the block, when it returns to the equilibrium position after being let go from rest at \(x = A\text{?}\)
Hint.
(a) When setting up forces, be mindful of the direction of forces from the two springs. (b) Compare with the undamped simple harmonic oscillator. (c) Use energy conservation; both springs have potential energies initially.
Answer.
(a) \(m a_x = -\left( k_1 +k_2 \right)\,x\text{,}\) (b) \(\omega = \sqrt{\dfrac{k_1 +k_2}{m}} \text{,}\) (c) \(\dfrac{1}{2}k_\text{eff} A^2\text{,}\) with \(k_\text{eff} = k_1 + k_2\text{.}\)
Solution 1. (a)
(a) Figure 13.46 shows that forces from both springs are in the same direction. Therefore, the \(x\) component of the net force on the block is
\begin{equation*} F_x = -k_1\,x -k_2\,x = -\left( k_1 +k_2 \right)\,x. \end{equation*}
Figure 13.46. Figure for Exercise 13.11.39(a) solution.
Therefore, equation of motion will be
\begin{equation*} m a_x = -\left( k_1 +k_2 \right)\,x. \end{equation*}
Dividing both sides by \(m\) we get
\begin{equation*} a_x = -\dfrac{k_1 +k_2}{m}\,x. \end{equation*}
Solution 2. (b)
(b) Compring the equation of motion to the equation of motion of simple harmonic motion, \(a_x=-\omega^2 x\text{,}\) we see that the angular frequency of the block here is
\begin{equation*} \omega^2 = \dfrac{k_1 +k_2}{m}, \end{equation*}
which can be written as
\begin{equation*} \omega^2 = \omega_1^2 + \omega_2^2, \end{equation*}
with
\begin{equation*} \omega_1^2 = \dfrac{k_1}{m},\ \text{ and }\ \omega_2^2 = \dfrac{k_2}{m}. \end{equation*}
Solution 3. (c)
From the conservation of energy, we note that kinetic energy at equilibrium will equal the potential energy at the end points of the motion.
\begin{equation*} KE = \dfrac{1}{2}k_\text{eff} A^2, \end{equation*}
where \(k_\text{eff} \) will be
\begin{equation*} k_\text{eff} = k_1 + k_2, \end{equation*}
based on \(\omega^2 = \dfrac{k_1 +k_2}{m} = \dfrac{k_\text{eff}}{m}\text{.}\)
40. A Block Attached to Two Springs in Series.
Two springs of spring constants \(k_1 \) and \(k_2\) are glued with a light but strong glue. One end of the combination is attached to a fixed wall and a block of mass \(m \) is attached to the other end and the block is placed on a frictionless table as shown in Figure 13.47.
Figure 13.47. Figure for Exercise 13.11.40.
(a) Show that the angular frequency of the block is given by
\begin{equation*} \omega = \sqrt{\dfrac{k_\text{eff} }{m}}, \end{equation*}
with
\begin{equation*} k_\text{eff} = \dfrac{k_1 k_2}{k_1 + k_2}. \end{equation*}
(b) Suppose the block is pulled a distance \(A \) from equilibrium, stretching the two springs in the process, and then released from rest. How fast is the block moving when it returns to the equilibrium position?
Hint.
(a) For displacement \(x\) of the block, springs have different stretches, say \(\Delta x_1\) and \(\Delta x_2\text{.}\) (b) Think conservation of energy with effective spring constant.
Answer.
(a) See solution, (b) \(\dfrac{1}{2}k_\text{eff}A^2 \text{.}\)
Solution 1. (a)
(a) Let \(x\) be the displacement of the block from its equilibrium position. Let \(\Delta x_1\) and \(\Delta x_2\) be the stretchings of the two springs at the instant shown in Figure 13.48. Therefore, we have
\begin{equation*} x = \Delta x_1 + \Delta x_2. \end{equation*}
We can write the equations of motions of the block and point P between the two springs.
\begin{align*} \amp \text{Block: }\ \ m a_x = -k_2\,\Delta x_2,\\ \amp \text{Point P: }\ \ k_1\Delta x_1 = k_2\,\Delta x_2, \end{align*}
Figure 13.48. Figure for Exercise 13.11.40(a) solution.
Eliminating \(\Delta x_1\) and \(\Delta x_2\) from the three equations above, we find
\begin{equation*} m a_x = - \dfrac{k_1k_2}{k_1 + k_2}\,x, \end{equation*}
which is an equation of a simple harmonic oscillator with effective spring constant \(ma_x = - k_\text{eff}\, x\text{,}\) with
\begin{equation*} k_\text{eff} = \dfrac{k_1k_2}{k_1 + k_2}. \end{equation*}
Making use of analogy with the simple harmonic motion we have the angular frequency of the block here
\begin{equation*} \omega = \sqrt{\dfrac{ k_\text{eff} }{m}}. \end{equation*}
Solution 2. (b)
(b) The potential energy stored is
\begin{equation*} U = \dfrac{1}{2}k_1 (\Delta x_1)^2 + \dfrac{1}{2}k_2 (\Delta x_2)^2, \end{equation*}
with
\begin{equation*} \Delta x_1 + \Delta x_2 = A \end{equation*}
andd
\begin{equation*} k_1 \Delta x_1 = k_2 \Delta x_2. \end{equation*}
From the last two equations, we get
\begin{equation*} \Delta x_1 = \dfrac{k_2}{k_1 + k_2}\, A,\ \ \ \Delta x_2 = \dfrac{k_1}{k_1 + k_2}\, A. \end{equation*}
Using these in \(U\) we get
\begin{equation*} U = \dfrac{1}{2}\,k_\text{eff}\, A^2. \end{equation*}
This will be the kinetic energy when block is at equilibrium since at that instant the potential energy will be zero and all energy will be the kinetic energy.
41. Disk Torsion Pedulum.
A disk of mass \(m\) and radius \(R\) is suspended from a thin string of torsion constant \(k\text{.}\) The string is fixed to the center of disk and oriented perpendicular to the disk. When the disk is rotated by a small angle about the equilibrium, there is a restoring torque due to twist in the string, which tends to bring the disk back to the equilibrium. Find the frequency of small oscillations about the equilibrium.
Solution.
The equation of motion of the rotation of the disk takes the form
\begin{equation*} I \ddot \theta = - k \theta,\ \textrm{with}\ I = \frac{1}{2} MR^2. \end{equation*}
This equation is analogous to \(m\ddot x = - k x\text{.}\) Therefore, the angular frequency \(\omega\) of oscillations will be
\begin{equation*} \omega = \sqrt{\frac{k}{I}} = \sqrt{\frac{2k}{MR^2}}. \end{equation*}
The frequency will be
\begin{equation*} f = \frac{\omega}{2\pi} = \frac{1}{2\pi R}\sqrt{\frac{2k}{M}} \end{equation*}
42. Car Shaking When Driven over Bumps Separated at Regular Distances.
The front suspension of a car has a natural frequency of 0.5 Hz. At the time the car was built, according to specifications, the shock absorbers of the car provide critical damping. With time, the shock absorbers get worn out so that they no longer provide the critical damping. The car then acts as an under-damped oscillator: when the car goes over a bump, it oscillates through many cycles. From measurements on the damped oscillations of the car, you calculate that \(\beta =\) 0.4 rad/sec. When the car is driven on a bumpy road with bumps placed at regular intervals of 50 m, the car shakes violently when driven at a particular speed. Find this critical speed that the driver must avoid.
Answer.
\(v = 24.8\) m/s.
Solution.
Let us write the given information first. The resonance frequency is given in terms of the distance and speed when the resonance occurs.
\begin{align*} \amp \omega_0 = 2\pi\times 0.5 = \pi\ \textrm{rad/s}.\\ \amp \beta = 0.4 \ \textrm{rad/s}.\\ \amp \textrm{Resonance Frequency},\ \ f_R = \frac{v}{D} = \frac{v}{50\ \textrm{m}}.\\ \amp \textrm{Resonance Angular Frequency},\ \ \omega_R = 2\pi f_R. \end{align*}
Now, using \(\omega_R = \sqrt{\omega^2 - 2\beta^2}\) we get the relation which can be used to find \(v\text{.}\)
\begin{equation*} 2\pi \frac{v}{50} = \sqrt{\pi^2 - 0.4^2}. \end{equation*}
Therefore, \(v = 24.8\) m/s.
PrevTopNext
Found typo?