Torsion Pendulum

Section 13.6 Torsion Pendulum

A torsion pendulum consists of a solid, a dumbbell, a disk, a bar, or an object of any other shape, suspended by a torsion wire from a fixed support as shown in Figure 13.23. A torsion wire is essentially a wire that could be twisted easily about its length. The twisting of the wire applies a restoring torque on the supported body whose tendency is to bring the body back to the configuration when the wire was not twisted, i.e., to the equilibrium.

According to the Hooke’s law for elasticity of materials, the restoring torque \(\tau\) on the bar should be proportional to the angle of twist, at least for the small angles of twist we would work with. Using a coordinate system in which the \(z\)-axis is pointed in the direction of the axis of rotation, the \(z\)-component of the torque would be

\begin{equation*} \tau_z= -\kappa\,\theta, \end{equation*}

where \(\kappa\) (read: kappa) is the torsional constant of the wire, and \(\theta\) is the \(z\)-component of the angular displacement from equilibrium as measured from \(x\)-axis in the \(xy\)-plane. The torsional constant for twisting wire is analogous to the spring constant of a spring. The rotational motion of the bar is then given by the \(z\)-component of the rotational equation of motion.

\begin{equation*} I_{zz}\frac{d^2\theta}{dt^2} = -\kappa\,\theta, \end{equation*}

where \(I_{zz}\) is the moment of inertia of the bar about \(z\)-axis, which is the axis of rotation. This equation is analogous to the equation for a plane pendulum in small angle approximation. By exploring the mathematical correspondence of symbols in the equations here to the equations of the simple pendulum we can read off the expression for the angular frequency \(\omega\) of oscillating motion of the torsion pendulum as

\begin{equation*} \omega = \sqrt{\frac{\kappa}{I_{zz}}}. \end{equation*}

Torsion pendulums are often used for time-keeping purposes, e.g., in the balance wheel of a mechanical watch. A torsion pendulum is also used in Cavendish experiment for determining the value of the Newton’s gravitational constant \(G_N\)

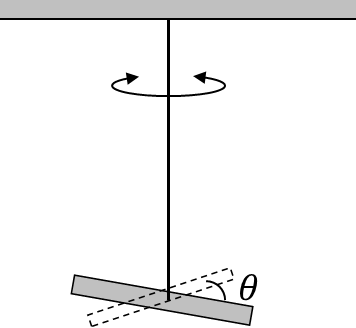
Example 13.24. Torsion Constant of a Torsion Wire.

A thin copper wire is tied to the center of a rod of mass \(100\ g\) and length \(20\ cm\) and hung from a fixed platform. When the copper wire is twisted and let go, the rod rotates about the wire with a time period of \(10\) seconds. What is the value of the torsion constant of the copper wire? Assume the moment of inertia of the wire itself about the axis of rotation to be small compared to that of the rod.

Solution.

From the give time period, we will find the angular frequency, and from the given geometry and masses, we will find the moment of inertia. Then we will use the formula for angular frequency to find the torsion constant of the wire.

Angular frequency:

\begin{equation*} \omega = \frac{2\pi}{T} = \frac{2\pi}{10\ sec} = 0.63\ rad/sec. \end{equation*}

Moment of inertia:

\begin{equation*} I = \frac{1}{12}m_{rod}l_{rod}^2 = 3.3\times 10^{-4}kg\ m^2. \end{equation*}

Therefore,

\begin{equation*} \kappa = I\,\omega^2 = 1.3\times 10^{-4}\ N.m. \end{equation*}

Prev Top Next