Momentum

Section 7.1 Momentum

Subsection 7.1.1 Definition of Momentum

The product of mass (\(m\)) and velocity (\(\vec v\)) is called momentum. We denote momentum vector by \(\vec p \) and the magnitude by the same letter without \(p\) the arrow.

\begin{equation} \vec p = m\, \vec v.\tag{7.1} \end{equation}

Momentum is a vector, just like velocity is a vector. The magnitude of momentum, denoted by the same symbol but without the overhead arrow, viz., \(p\text{,}\) is obtained by multiplying the mass and speed \(v\text{,}\) and the direction of momentum is same as the direction of velocity.

\begin{align*} \amp \text{Magnitude, } p = m\, v,\\ \amp \text{Direction of } \vec p \text{ is same as the direction of } \vec v. \end{align*}

You can also think of mementum in terms of its Cartesian components, similar to other vectors. Thus, \(x \text{,}\) \(y \) and \(z \) components of momentum will be equal to the product of mass and corresponding component of the velocity vector.

\begin{align*} \amp p_x = m\, v_x,\\ \amp p_y = m\, v_y,\\ \amp p_z = m\, v_z. \end{align*}

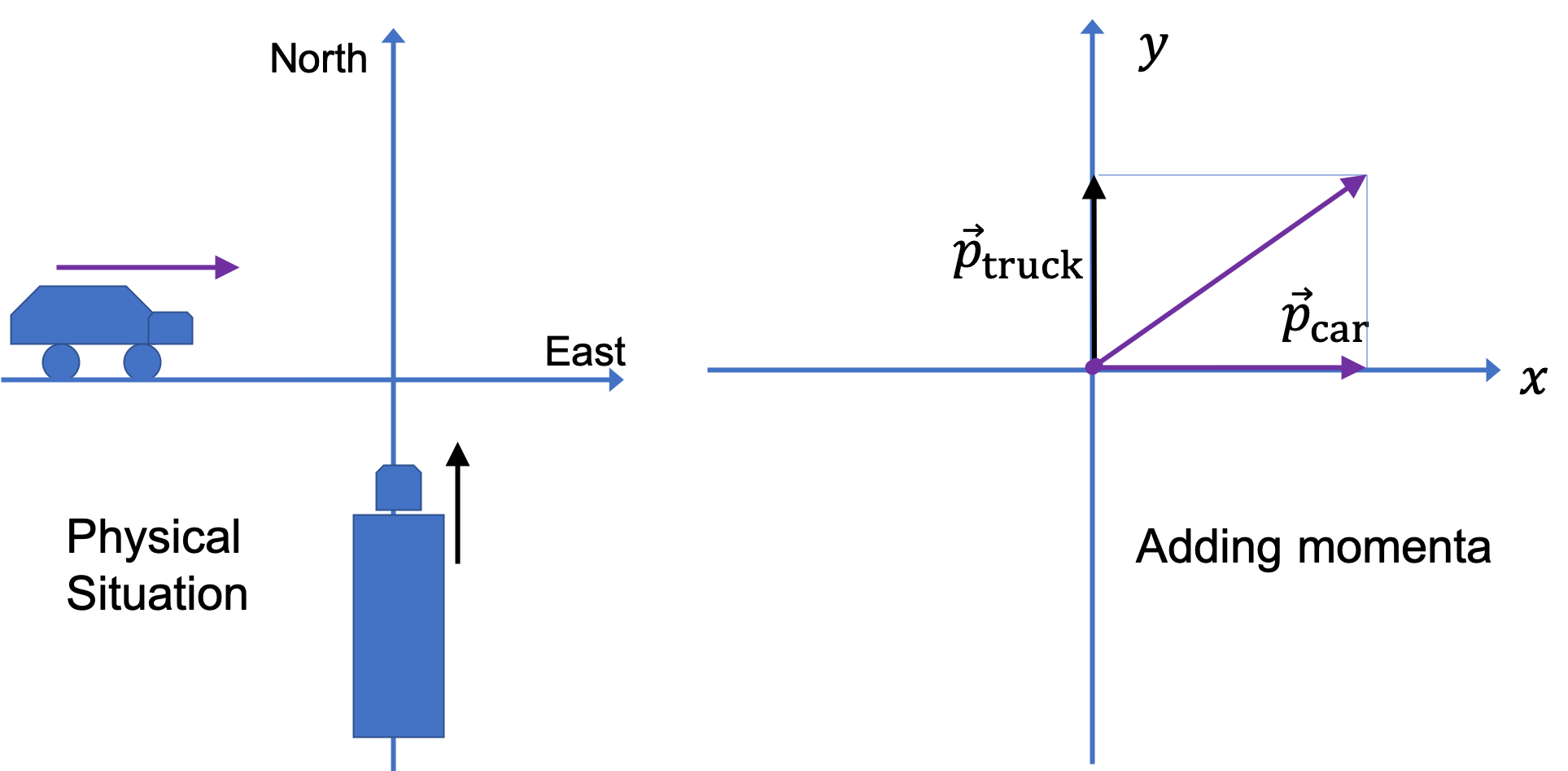
Example 7.1. Momentum of One Body.

A car of mass \(3000\text{ kg}\) is moving to the East at a speed of \(3\text{ m/s}\text{.}\)

(a) How much momentum does the car have?

(b) If you ride your motorcycle, with what speed must you ride to equal the same momentum? Assume motorcycle plus your mass is \(300\text{ kg}\text{.}\)

Answer.

(a) \(9,000 \text{ kg.m/s} \text{,}\) East, (b) \(30\text{ m/s}\text{,}\) East.

Solution 1. a

It would be a mistake to just multiply mass with the speed and report that as the momentum since momentum is a vector quantity. Therefore, we must indicate both the magnitude and direction in our answer.

\begin{align*} \amp\text{Magnitude } = mv = (3000\ \text{ kg}) \times (3\ \text{ m/s}) = 9,000 \text{ kg.m/s}.\\ \amp\text{Direction: Towards East.} \end{align*}

Solution 2. b

Since mass is \(10 \times\) less, you will need to ride at speed \(10 \times\) greater to have the same magnitude of momentum.

\begin{equation*} v = 10\times 3 = 30\text{ m/s}. \end{equation*}

You could also set up an equation.

\begin{equation*} m_\text{mc}v_\text{mc} = m_\text{car}v_\text{car}. \end{equation*}

Of course, your direction will also have to match that of the car for the momentum to be truly equal.

Subsection 7.1.2 Momentum is Relative

Since velocity is defined relative to an observer, momentum is also relative to an observer. For instance, say, you and your friend are sitting in a train which is moving with respect to a ground-based observer. Then, your friend’s momentum with respect to you will be zero but non-zero with respect to the ground-based observer.

Figure 7.2 shows a particle of mass \(m\) that moves with velocity \(\vec v\) as observed from reference point O. A person at O’ has velocity \(\vec V\) with respect to O. The velocity of the particle observed by O’ will be

\begin{equation*} \vec v' = \vec v - \vec V. \end{equation*}

Therefore, momentum of the particle observed by person O’, say \(\vec p'\) will be different than the momentum, say \(\vec p\text{,}\) observed with respect to O.

\begin{equation*} \vec p' = \vec p - m\vec V. \end{equation*}

Example 7.3. Momentum of a Cannon Ball Fired From a Boat on a River.

A cannon ball of mass \(20\text{ kg}\) is fired from a boat on a river at a speed of \(40\text{ m/s}\) with respect to the boat at an angle \(30^\circ\) from the ground towards the East direction. The boat itself is going at a speed of \(10\text{ m/s}\) with respect to the shore towards North on the river. (a) What is the momentum of the cannon ball with respect to the boat? (b) What is the momentum of the cannon ball with respect to the shore?

Answer.

(a) \(800\text{ kg.m/s}\) at \(30^\circ\) above horizon towards East. (b) \(825\text{ kg.m/s}\) at angles to be figured out by the student.

Solution 1. a

The velocity with respect to the boat in components is

\begin{equation*} \vec v = 40\,\cos\,30^\circ \hat i + 40\,\sin\,30^\circ \hat k. \end{equation*}

Therefore, momentum of the ball with respect to the boat is (units \(\text{kg.m/s}\))

\begin{equation*} m\vec v = 800\,\cos\,30^\circ \hat i + 800\,\sin\,30^\circ \hat k. \end{equation*}

The magnitude is \(800\text{ kg.m/s}\text{,}\) and direction same as direction of this velocity.

Solution 2. b

In Figure 7.4 we have \(y\)-axis to the North. Therefore, the velocity of the boat with respect to the shore is

\begin{equation*} \vec V = 10\,\hat j. \end{equation*}

Now, we add this to the velocity of the ball with respect to the boat to get the velocity of the ball with respet to the shore. Let us denote this velocity with \(\vec w\text{.}\)

\begin{equation*} \vec w = \vec V + \vec v = 40\,\cos\,30^\circ \hat i + 10\,\hat j + 40\,\sin\,30^\circ \hat k. \end{equation*}

From this we get momentum of the cannon ball with respect to the shore to be

\begin{equation*} m \vec w = 800\,\cos\,30^\circ \hat i + 200\,\hat j + 800\,\sin\,30^\circ \hat k. \end{equation*}

You can get the magnitude by

\begin{equation*} \sqrt{800^2\cos^2\,30^\circ + 200^2 + 800^2\sin^2\,30^\circ} = 825\text{ kg.m/s}. \end{equation*}

For direction, you can figure out the spherical coordinate angle. That is left as an exercise.

Subsection 7.1.3 Total Momentum of a Multi-part System

If a system consists of more than one part, then the momentum of the whole will be equal to the vector sum of the momenta of all of its parts.

Figure 7.5 shows a system of two particles connected by a light rod. Ignoring the momentum of the rod, the total momentum of the two particles will be just a vector sum.

\begin{equation} \vec p_{\text{net}} = m_1\vec v_1 + m_2\vec v_2.\tag{7.2} \end{equation}

Example 7.6. Adding Momenta.

Figure 7.7 shows a system of two particles. Their individual momenta written as vector using Cartesian unit vectors are

\begin{gather*} \vec p_1 = 6\text{ kg m/s}\;\hat j.\\ \vec p_2 = -6\text{ kg m/s}\;\hat i. \end{gather*}

Their sum will be

\begin{equation*} \vec p_{\text{net}} = 6\text{ kg m/s}\;(-\hat i + \hat j) \end{equation*}

Therefore, magnitude will

\begin{equation*} p_{\text{net}} = \sqrt{ 6^2 + 6^2 } = 6\sqrt{2}\; \text{ kg m/s}, \end{equation*}

and direction will be obtained by arctan

\begin{equation*} \theta = \tan^{-1}(-1/1)= - 45^\circ. \end{equation*}

Since \(\vec p_{\text{net}}\) is pointed in the second quadrant, this angle is clockwise from negative \(x\) axis.

In general, let \(\vec p_1\text{,}\) \(\vec p_2 \text{,}\) \(\cdots\text{,}\) \(\vec p_N\) be the momenta of \(N \) parts of a system, then the momentum of the whole, the net momentum is the vector sum of the parts.

\begin{equation} \vec p_{\text{net}} = \vec p_1 + \vec p_2 + \cdots + \vec p_N. \tag{7.3} \end{equation}

Note that the sum of the momenta is a vector sum and one must be careful not to just add the magnitudes of the momenta since directions are equally important.

Another aspect of adding momenta is to note that the momenta on the right side of formula in Eq. (7.3) are all with respect to the same reference point.

Example 7.8. Same reference for all momenta to be added.

For instance, if you (mass \(m\)) are walking on a truck with a velocity \(\vec v\) with respect to the platform. And truck (of mass \(M\)) is moving with respect to the ground with velocity \(\vec V\text{.}\) Then total momentum of you and the truck with respect to the ground will be

\begin{equation*} \vec p_\text{net} = M\vec V +m (\vec v + \vec V). \end{equation*}

This is clearly not simply \((M\vec V + m \vec v)\text{.}\) The additional term comes from expressing your momentum with respect to the ground and not with respect to the truck.

Exercises 7.1.4 Exercises

1. Components of Momentum Vector in a Plane.

The momentum of a runner is \(400\text{ kg.m/s}\) in the direction \(30^{\circ}\) clockwise with respect to the positive \(x\) axis in the \(xy\)-plane as shown in Figure 7.10. What are the \(x\) and \(y\) components of the momentum?

Hint.

Drop projections on the \(x \) and \(y\) axes.

Answer.

\(p_x = 346 \text{ kg.m/s}\text{,}\) \(p_y = -200 \text{ kg.m/s}\text{.}\)

Solution.

From the projections on \(x \) and \(y\) axes, we know that \(p_x\gt 0\) and \(p_y \lt 0\text{.}\)

\begin{align*} \amp p_x = 400\, \cos\, 30^{\circ} = 346\, \text{ kg.m/s}. \\ \amp p_y = -400\, \sin\, 30^{\circ} = -200\, \text{ kg.m/s}. \end{align*}

2. Total Momentum of Two Bodies Moving on the Same Line.

A car of mass \(3000\text{ kg}\) is moving to the East at a speed of \(30\text{ m/s}\) and a truck of mass \(6000\text{ kg}\) is moving to the West at a speed of \(20\text{ m/s}\text{.}\) What is the net momentum of the two?

Hint.

Be mindful of the vector nature of momentum.

Answer.

\(30,000\text{ kg.m/s}\text{,}\) West.

Solution.

By applying the definition of momentum to the car and the truck separately, we find that the car has a momentum of \(90,000\text{ kg.m/s}\) pointed towards East and the truck has a momentum of \(120,000\text{ kg.m/s}\) pointed towards West. Now, we need to add these two vectors. Since their directions are opposite, smaller magnitude will be subtracted from the larger magnitude, resulting in a net momentum of \(30,000\text{ kg.m/s}\) pointed towards the larger momentum direction, which is West in our case.

Analytic Method: You can also use the analytic method to add the two vectors by utilizing a Cartesian coordinate. To be concrete, let us point the \(x \) axis towards the East. Then, the two momentum vectors will have the following \(x \) components (the \(y \) and \(z \) components of both being zero).

\begin{align*} \amp p_{\text{car},x} = 90,000\ \text{kg.m/s}, \\ \amp p_{\text{truck},x} = - 120,000\ \text{kg.m/s}, \end{align*}

where \(p_{\text{truck},x} \) is negative since \(\vec p_{\text{truck}} \) is pointed towards the negative \(x \) axis. Therefore, the net momentum has the following \(x \) component (the \(y \) and \(z \) components of both being zero).

\begin{align*} \amp p_{\text{net},x} = -30,000\ \text{kg.m/s} \end{align*}

Therefore, the net momentum has the magnitude \(30,000\text{ kg.m/s}\) pointed towards the negative \(x \) axis, which is towards the West.

3. Total Momentum of Two Bodies Moving in a Plane.

A car of mass \(3000\text{ kg} \) is moving East at a speed of \(30\text{ m/s} \) and a truck of mass \(6000\text{ kg} \) is moving North at a speed of \(20\text{ m/s} \text{.}\) What is the net momentum of the two?

Hint.

Be mindful of the vector nature of momentum.

Answer.

\(150,000\text{ kg.m/s}, 53^{\circ} \) North of East.

Solution.

Now, we need to add these two vectors. Since the two vectors are not along one line, we need to employ the parallelogram law of addition of vectors, either geometrically or analytically. We have seen that analytical method is often easier to implement.

Figure 7.11. Figure for Exercise 7.1.4.3.

To use the analytical method, as usual we start with a choice of coordinate system as shown in Figure 7.11. Let us point the \(x \) axis towards East and the \(y \) axis towards North. The \(z \) axis is pointed vertically up, but we ignore it in this problem since the vectors are only in the horizontal plane. Then, we see that the two momenta have the following representation in components.

\begin{align*} \amp \vec p_{\text{car}} = \left( 90,000\ \text{ kg.m/s},0,0\right)\\ \amp \vec p_{\text{truck}} = \left( 0, 120,000\ \text{ kg.m/s},0 \right) \end{align*}

where \(x\text{,}\) \(y\) and \(z\) components of each vector have been listed in order. Therefore, the net momentum has the following representation in the given coordinate system.

\begin{equation*} \vec p_{\text{net}} = \left( 90,000\text{ kg.m/s}, 120000\text{ kg.m/s},0 \right). \end{equation*}

To find the magnitude and direction of the net momentum, we just need to implement the components to (magnitude, direction) transformation. In the \(xy\)-plane, we need only one angle for the direction.

\begin{align*} \amp \text{Magnitude, }p_{\text{net}} = \sqrt{90000^2 + 120000^2 } = 150,000\text{ kg.m/s},\\ \amp \text{Direction, }\theta = \arctan( 120000/90000) = 53^{\circ}, \end{align*}

where the angle is counterclockwise with respect to the positive \(x \) axis, which gives the direction of \(53^{\circ} \) North of East.

Prev Top Next