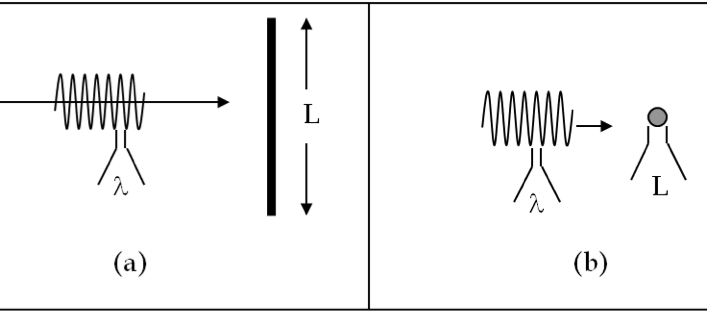
1. Deciding Between Wave Optics and Ray Optics.
Decide when you will have to use wave optics in the following situations.
(a) A green laser light of wavelength \(530\text{ nm}\) is incident on a mirror of size \(30\text{ cm}\text{.}\) Will your answer change if you also needed to fidn intensity of the reflected and transmitted waves?
(b) A red laser light of wavelength \(632\text{ nm}\) is incident on a bead of diameter \(10\:\mu\text{m}\text{.}\) Will your answer change if you also needed to fidn intensity of the reflected and transmitted waves?
(c) A radio wave of frequency \(10^9\text{ Hz}\) is incident on a square aluminum plate of area \(9 \text{m}^2\text{.}\) Will your answer change if you also needed to fidn intensity of the reflected and transmitted waves?
(d) A radio wave of frequency \(10^9\text{ Hz}\) incident on a square aluminum plate of area \(9\text{ cm}^2\text{.}\) Will your answer change if you also needed to fidn intensity of the reflected and transmitted waves?
Hint.
Compare wavelength with the dimension of the object.
Answer.
(a) Ray, (b) Wave, (c) Ray, (d) Wave. If intensity required, we need to study wave picture.
Solution 1. (a)
Here dimension is \(L=30\text{ cm}\) and wavelength \(\lambda = 530\text{ nm}\text{.}\) These give us
\begin{equation*}
\frac{L}{\lambda} = 5.66\times 10^5.
\end{equation*}
That is \(L \gt\gt \lambda\text{.}\) We will use ray optics here if we do not need to study intensity.
Yes, the answer will change since for intensity studies we need the wave picture.
Solution 2. (b)
Here dimension is \(L=2.0\:\mu\text{m}\) and wavelength \(\lambda = 632\text{ nm}\text{.}\) These give us
\begin{equation*}
\frac{L}{\lambda} = 3.16.
\end{equation*}
That is \(L \sim \lambda\text{.}\) We must use wave optics here.
No, the answer will not change since for intensity studies we need the wave picture.
Solution 3. (c)
Compare \(\lambda\) to the smallest dimensions of the plate, which would be the square-root of the area, \(\sqrt{A}\text{.}\) Note, we cannot compare a length to an area, that is why we must get the square root of the area. Here
\begin{align*}
\amp \lambda = \frac{3\times 10^8\:\textrm{m/s}}{10^9\:\textrm{Hz}} = 0.3\:\textrm{m}\\
\amp \sqrt{A} = 3 \text{ m}
\end{align*}
Therefore, we have \(\lambda \lt \lt \sqrt{A}\text{,}\) we can use the ray approximation if we do not need intensity studies.
Yes, the answer will change since for intensity studies we need the wave picture.
Solution 4. (d)
Here, \(\sqrt{A}= 0.03\text{ m}\) and \(\lambda = 0.3\text{ m}\text{.}\) Since \(\lambda \gt \gt \sqrt{A}\text{,}\) we need to use the wave picture.
No, the answer will not change since for intensity studies we need the wave picture.